Question

Find the area of a parallelogram whose adjacent sides are given by the vectors
\[\overrightarrow{a}=3\widehat{i}+\widehat{j}+4\widehat{k}\] and \[\overrightarrow{b}=\widehat{i}-\widehat{j}+\widehat{k}\] .

Answer 1

Hint: The adjacent side vectors of the parallelogram ABCD are \[\overrightarrow{a}=3\widehat{i}+\widehat{j}+4\widehat{k}\] and \[\overrightarrow{b}=\widehat{i}-\widehat{j}+\widehat{k}\] . We know the formula that if the vectors \[\overrightarrow{a}\] and \[\overrightarrow{b}\] are the adjacent sides of a parallelogram then the area of the parallelogram is the magnitude vector product of the adjacent sides of the parallelogram i.e., The area of the parallelogram = \[\left| \overrightarrow{a}\times \overrightarrow{b} \right|\] . Use this formula and calculate the area vector. Now, get the magnitude of the area vector using the formula that magnitude of a vector \[xi+yj+zk\] is \[\sqrt{{{x}^{2}}+{{y}^{2}}+{{z}^{2}}}\] .

Complete step by step answer:
According to the question, we are given the adjacent sides of a parallelogram in vector form and we are asked to find the area of the parallelogram.
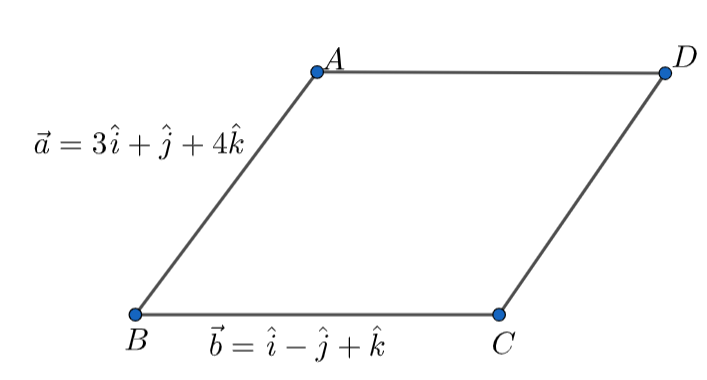
The vector form of side AB of the parallelogram is \[\overrightarrow{a}=3\widehat{i}+\widehat{j}+4\widehat{k}\] ……………………………………..(1)
The vector form of side BC of the parallelogram is \[\overrightarrow{b}=\widehat{i}-\widehat{j}+\widehat{k}\] ……………………………………..(2)
Here, we have to find the area of the parallelogram.
We know the formula that if the vectors \[\overrightarrow{a}\] and \[\overrightarrow{b}\] are the adjacent sides of a parallelogram then the area of the parallelogram is the magnitude vector product of the adjacent sides of the parallelogram i.e., The area of the parallelogram = \[\left| \overrightarrow{a}\times \overrightarrow{b} \right|\] …………………………………..(3)

Now, from equation (1), equation (2), and equation (3), we get
The area of the parallelogram = \[\left[ \left( 3\widehat{i}+\widehat{j}+4\widehat{k} \right)\times \left( \widehat{i}-\widehat{j}+\widehat{k} \right) \right]\] ………………………………………..(4)
We know the property that \[\widehat{i}\times \widehat{i}=0\] , \[\widehat{j}\times \widehat{j}=0\] , \[\widehat{k}\times \widehat{k}=0\] , \[\widehat{i}\times \widehat{j}=\widehat{k}\] , \[\widehat{i}\times \widehat{k}=-\widehat{j}\] , \[\widehat{j}\times \widehat{i}=-\widehat{k}\] ,
\[\widehat{j}\times \widehat{k}=\widehat{i}\] , \[\widehat{k}\times \widehat{i}=\widehat{j}\] , and \[\widehat{k}\times \widehat{j}=\widehat{-i}\] ……………………………………..(5)
Now, from equation (4) and equation (5), we get
The area of the parallelogram ABCD = \[\left[ \left( 3\widehat{i}+\widehat{j}+4\widehat{k} \right)\times \left( \widehat{i}-\widehat{j}+\widehat{k} \right) \right]\] = \[\left( 5\widehat{i}+\widehat{j}-4\widehat{k} \right)\] ……………………………………..(6)
We know the formula for the magnitude of a vector \[xi+yj+zk\] , Magnitude = \[\sqrt{{{x}^{2}}+{{y}^{2}}+{{z}^{2}}}\] …………………………………………………(7)
Now, from equation (6) and equation (7), we get
The area of the parallelogram = \[\sqrt{{{5}^{2}}+{{1}^{2}}+{{\left( -4 \right)}^{2}}}=\sqrt{25+1+16}=\sqrt{42}\] .
Therefore, the area of the parallelogram is \[\sqrt{42}\] sq. units.

Note:
 We can also solve this question using the matrix method formula that is, the area of the parallelogram whose adjacent side vectors are \[{{x}_{1}}\widehat{i}+{{y}_{1}}\widehat{j}+{{z}_{1}}\widehat{k}\] and \[{{x}_{2}}\widehat{i}+{{y}_{2}}\widehat{j}+{{z}_{2}}\widehat{k}\] is given by \[\left| \begin{align}
  & \begin{matrix}
   \widehat{i} & \,\,\,\widehat{j} & \widehat{\,k} \\
\end{matrix} \\
 & \begin{matrix}
   {{x}_{1}} & {{y}_{1}} & {{z}_{1}} \\
\end{matrix} \\
 & \begin{matrix}
   {{x}_{2}} & {{y}_{2}} & {{z}_{2}} \\
\end{matrix} \\
\end{align} \right|\] .