Question

How do you find the area of a regular octagon inscribed in a circle whose equation is given by ${{\left( x-2 \right)}^{2}}+{{\left( y+3 \right)}^{2}}=25$?

Answer 1

Hint: An octagon is a polygon with $8$ sides. But if it is a regular octagon, all it’s sides are of the same length. So here the octagon is inscribed in the circle where all it’s vertices are lying on the circle. We know that the central angle made by the entire circle is ${{360}^{\circ }}$. And when the radius is joined with the vertices of the octagon, we can see that $8$ triangles with the same side length and same angles are formed. Let us make use of properties of triangles to solve further.

Complete step by step solution:
Picture for reference :

Let us first find the center of the circle and its radius. To get them, we will just compare the given circle with the standard equation of the circle.
The standard equation of the circle is : ${{\left( x-a \right)}^{2}}+{{\left( y-b \right)}^{2}}={{r}^{2}}$ where $\left( a,b \right)$ is the coordinate of the center and $r$is the radius.
Upon comparing ${{\left( x-2 \right)}^{2}}+{{\left( y+3 \right)}^{2}}=25$ with the standard equation , the center of the circle is $C\left( 2,-3 \right)$ which is denoted by $C$. And the radius is $5$.
We already know that the central angle made by the entire circle is ${{360}^{\circ }}$. After we join the radius with the vertices of the octagon, we can see that $8$ triangles with the same side length and same angles are formed. The central angle is being equally divided into $8$ parts. And each central angle fragment is the top angle of the triangle formed.
The top angle of each triangle formed is equal to $\dfrac{{{360}^{\circ }}}{8}={{45}^{\circ }}$.
The length of two sides of the triangle formed is the same since it is the radius of the circle. And we know one angle which is ${{45}^{\circ }}$.
So now let us focus on the triangle $\Delta CGF$. We can find the area of this triangle and then multiply it by $8$ to find the area of the octagon since all the triangles are identical.

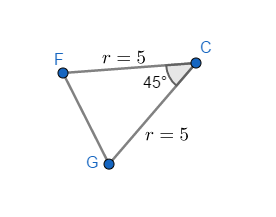
To find out the area of this triangle, let us make use of formulas from properties of triangles.
The formula to find the area of a triangle when two of its sides and the angle made by those sides are known is $\dfrac{1}{2}ab\sin C$ where $a,b$ are the lengths of the side and $C$ is the angle made by them.
Here $a=FC=5,b=GC=5$ and $\angle C={{45}^{\circ }}$
Let us substitute these values and solve for the area.
$ar\left( \Delta CGF \right)=\dfrac{1}{2}\times 5\times 5\times \sin {{45}^{\circ }}=\dfrac{25}{2}\times \dfrac{1}{\sqrt{2}}=\dfrac{25}{2\sqrt{2}}$ .
Area of the regular octagon is $8\times ar\left( \Delta CGF \right)=8\times \dfrac{25}{2\sqrt{2}}=50\sqrt{2}$.

$\therefore $ Hence, the area of a regular octagon inscribed in a circle whose equation is given by ${{\left( x-2 \right)}^{2}}+{{\left( y+3 \right)}^{2}}=25$ is $50\sqrt{2}$.

Note: It is very important to remember all the formulae of properties of triangle as they are highly useful and their usage is not just limited to trigonometry. Their concepts can even be used in calculus and geometry. It is also important to know the standard equation of the circles and other related formulae so as to solve the question quickly. We should be careful while comparing the given circle equation with the standard one to get the center correct or else our graph would be wrong and the results we infer from the graph would also be wrong.