Question

Find the area of a segment of a circle of radius 21cm, if the angle made by the arc of the segment has a measure of ${{60}^{\circ }}$?
\[\begin{align}
  & A.45.27c{{m}^{2}} \\
 & B.40.27c{{m}^{2}} \\
 & C.40.8c{{m}^{2}} \\
 & D.44.27c{{m}^{2}} \\
\end{align}\]

Answer 1

Hint: In this problem, we will first draw figures for better understanding. For finding the area of the segment subtended by angle ${{60}^{\circ }}$ we need to find the area of a sector of the circle subtended by ${{60}^{\circ }}$ and the area of the triangle formed by chord and radius of the circle as
Area of segment = area of the sector - area of the triangle. We will use the formula for finding the area of the sector given by $\dfrac{\theta }{360}\times \pi {{r}^{2}}$ where $\theta $ is the angle which subtends sector and segment and r is the radius of the circle. Area of triangle is given by $\dfrac{1}{2}\times \text{base}\times \text{height}$ where base and height will be found using congruence of triangle and $\sin \theta ,\cos \theta $ formula in right-angled triangle.

Complete step-by-step solution:
Let us draw the figure first:

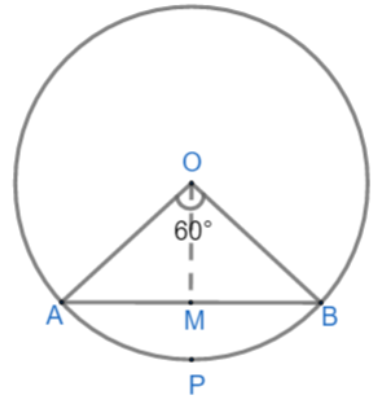
Here we are given angle as ${{60}^{\circ }}$. Therefore, $\theta ={{60}^{\circ }}$ As we can see from diagram, area of segment APB = area of sector OAPB - area of triangle OAB . . . . . . . . . . . . . . (1)
So, let us find the area of sector OAPB and the area of triangle OAB. Given radius r = 21cm.
\[\begin{align}
  & \text{Area of sector OAPB}=\dfrac{\theta }{360}\times \pi {{r}^{2}} \\
 & \text{Area of sector OAPB}=\dfrac{60}{360}\times \dfrac{22}{7}\times 21\times 21 \\
 & \text{Area of sector OAPB}=231c{{m}^{2}}\cdots \cdots \cdots \cdots \left( 2 \right) \\
\end{align}\]
\[\text{Area of triangle AOB}=\dfrac{1}{2}\times \text{base}\times \text{height}\]
But we don't know the value of base and height for triangle AOB.
Let us draw $OM\bot AB$ therefore, $\angle OMB=\angle OMA={{90}^{\circ }}$

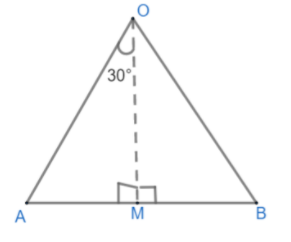
In $\Delta OMA\text{ and }\Delta OMB$ we can see that $\angle OMB=\angle OMA={{90}^{\circ }}$
OA = OB as both are the radius of the same circle.
OM = OM which is common in both triangles.
Therefore, by RHS congruence criterion, $\Delta OMA\simeq \Delta OMB$.
By using CPCT (corresponding parts of the congruent triangle) we can say that,
$\angle AOM=\angle BOM\text{ and BM=AM}$.
Therefore, $\angle AOM=\angle BOM=\dfrac{1}{2}\angle BOA$.
As we know $\angle BOA={{60}^{\circ }}$.
Therefore, $\angle AOM=\angle BOM=\dfrac{1}{2}\times {{60}^{\circ }}={{30}^{\circ }}$.
Also, $BM=AM=\dfrac{1}{2}AB$.
Now from $\Delta OMA$.
\[\begin{align}
  & \sin {{30}^{\circ }}=\dfrac{\text{Side opposite of angle }{{30}^{\circ }}}{\text{Hypotenuse}} \\
 & \Rightarrow \dfrac{1}{2}=\dfrac{AM}{AO} \\
 & \Rightarrow \dfrac{1}{2}=\dfrac{AM}{21} \\
 & \Rightarrow AM=\dfrac{21}{2} \\
\end{align}\]
Also,
\[\begin{align}
  & \cos {{30}^{\circ }}=\dfrac{\text{Side adjacent of angle }{{30}^{\circ }}}{\text{Hypotenuse}} \\
 & \Rightarrow \dfrac{\sqrt{3}}{2}=\dfrac{OM}{AO} \\
 & \Rightarrow \dfrac{\sqrt{3}}{2}=\dfrac{OM}{21} \\
 & \Rightarrow OM=\dfrac{\sqrt{3}}{2}\times 21 \\
\end{align}\]
As we have found AB = 2AM
Putting the value of AM, we get AB = 21cm.
Also, $OM=\dfrac{21\sqrt{3}}{2}cm$.
So we have found the value of base and height for triangle AOB. Hence,
\[\begin{align}
  & \text{Area of triangle AOB}=\dfrac{1}{2}\times \text{AB}\times \text{OM} \\
 & \Rightarrow \text{Area of triangle AOB}=\dfrac{1}{2}\times 21\times \dfrac{\sqrt{3}}{2}\times 21 \\
 & \Rightarrow \text{Area of triangle AOB}=\dfrac{441\sqrt{3}}{4}c{{m}^{2}} \\
\end{align}\]
From (1), Area of segment APB = area of sector OAPB - an area of triangle OAB.
Hence,
\[\begin{align}
  &\text{Area of segment APB}=\left( 231-\dfrac{441\sqrt{3}}{4} \right)c{{m}^{2}} \\
 &\Rightarrow 231-190.83c{{m}^{2}} \\
 &\Rightarrow 40.27c{{m}^{2}} \\
\end{align}\]
Thus, required area $40.27c{{m}^{2}}$.

Note: Students should always draw diagrams for these sums for better understanding. Don't forget to put units after finding the values. Area of triangle in this case can be found in the following ways:
Since one of the angle is \[{{60}^{\circ }}\] and it is an isosceles triangle (two of the sides are radius) therefore, $\angle OAB=\angle OBA$. By angle sum property ${{60}^{\circ }}+\angle OAB+\angle OBA={{180}^{\circ }}$. Hence, $\angle OAB=\angle OBA={{60}^{\circ }}$. Hence, it is an equilateral triangle. Therefore, area of triangle $\Rightarrow \dfrac{\sqrt{3}}{4}{{\left( \text{side} \right)}^{2}}=\dfrac{\sqrt{3}}{4}{{\left( 21 \right)}^{2}}=\dfrac{441\sqrt{3}}{4}c{{m}^{2}}$.