
Find the Cartesian equation of the line which passes through the point
Answer
500.7k+ views
Hint: To solve this problem, we should know the relation the directional ratios of two parallel lines. The corresponding directional ratios of two parallel lines are proportional. Mathematically, if two lines with directional ratios
Complete step by step answer:
We can infer from the question that the required line is parallel to the line
We know that the condition for two lines to be parallel is that the corresponding directional ratios of the two lines should be proportional which is mathematically written as two lines with directional ratios
The directional ratios of a line of the form
Let us consider the given line
We can rewrite the equation as
Comparing this equation with the above relation, we get the directional ratios of the line as
Let us consider the directional ratios of the required line be a, b, c respectively. We can write the relation between them from the condition of parallel lines as
We know that, if the directional ratios of a line are a, b, c and the line pass through the point
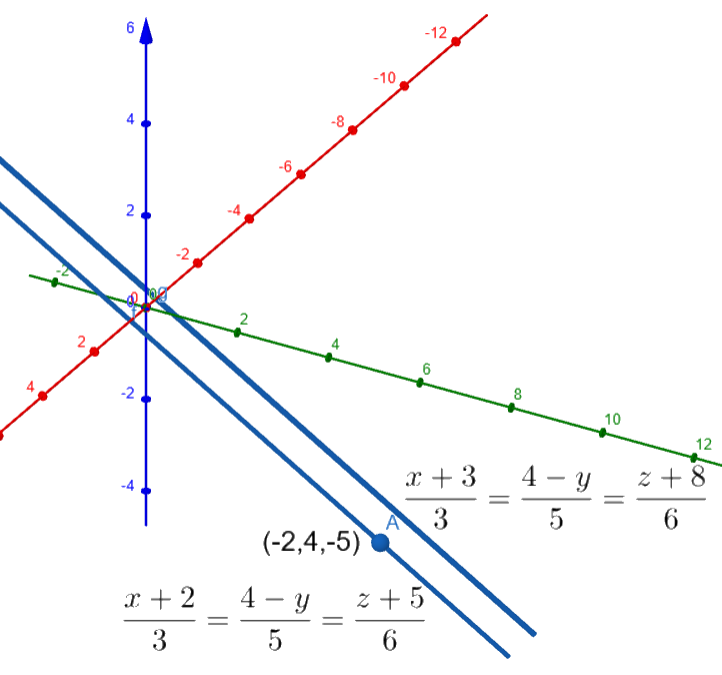
We can infer that the required line passes through the point A
From the above relation with
We get the equation of the line as
Cancelling k in all the three terms, we get
Note: Students might commit a mistake while writing the directional ratios of
Complete step by step answer:
We can infer from the question that the required line is parallel to the line
We know that the condition for two lines to be parallel is that the corresponding directional ratios of the two lines should be proportional which is mathematically written as two lines with directional ratios
The directional ratios of a line of the form
Let us consider the given line
We can rewrite the equation as
Comparing this equation with the above relation, we get the directional ratios of the line as
Let us consider the directional ratios of the required line be a, b, c respectively. We can write the relation between them from the condition of parallel lines as
We know that, if the directional ratios of a line are a, b, c and the line pass through the point
We can infer that the required line passes through the point A
From the above relation with
We get the equation of the line as
Cancelling k in all the three terms, we get

Note: Students might commit a mistake while writing the directional ratios of
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




