Question

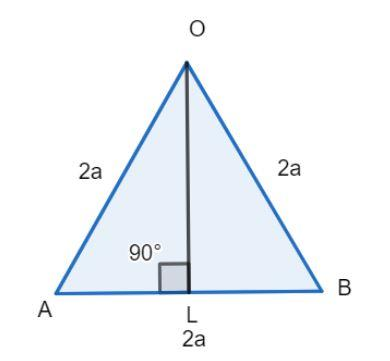
Find the coordinates of vertices of an equilateral triangle of side 2a as shown in the figure.

Answer 1

Hint: Find the length of the altitude OL. It is the y-coordinate of the vertices A and B. The x-coordinates can be found using the length of the side of the triangle.

Complete step-by-step answer:
It is given that the triangle OAB is an equilateral triangle of side 2a and we need to find the vertices of this triangle.
One of the vertices is already the origin and hence, given by O (0, 0).
OL is the altitude of the triangle OAB from the vertex O to the side AB. The altitudes of an equilateral triangle are the perpendicular bisectors of the sides.
Hence, AL is equal to LB and the length is half of the side AB, which is a.
Hence OL is perpendicular to AB, then, the triangle OLB is a right-angled triangle.
Using Pythagoras theorem to the sides of the triangle OLB, we have:
\[O{L^2} + L{B^2} = O{B^2}\]
Simplifying, we have:
\[O{L^2} + {a^2} = {(2a)^2}\]
Solving for OL, we have:
\[O{L^2} + {a^2} = 4{a^2}\]
\[O{L^2} = 4{a^2} - {a^2}\]
\[O{L^2} = 3{a^2}\]
Taking square root on both sides, we have:
\[OL = \sqrt 3 a\]
Hence, the coordinates of the point L is \[(0,\sqrt 3 a)\].
Point A is a unit in the negative x-direction of point L and hence, the coordinates of A are \[( - a,\sqrt 3 a)\].
Point B is a unit in the positive x-direction of point L and hence, the coordinates of B are \[(a,\sqrt 3 a)\].
Hence, the coordinates of the vertices of the triangle are O (0, 0), A \[( - a,\sqrt 3 a)\], and B \[(a,\sqrt 3 a)\].

Note: It is not explicitly given that O is origin but in general the point O represents the origin which has coordinates (0, 0).