
Find the electric field at the center of an arc of linear charge density

Answer
426.6k+ views
1 likes
Hint: First of all consider small corresponding elements of arc on either side of the horizontal. Now find out the electric field produced due to them and the resolve and also cancel out the oppositely directed ones. Now substitute the standard expression for electric field due to charge density
Formula used:
Electric field,
Complete step-by-step solution
We are given an arc of linear charge density
The arc is subtending angle
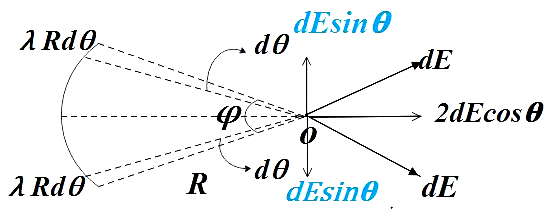
Now resolving the electric field due to small element of the arc
So we have
We know that electric due to charge dQ is given by,
But,
Substituting (2) in (3),
Now substituting the value of K as
Hence, we find the electric field at the centre of an arc of linear charge density
Note: If the given arc subtended
Similarly, you could find the electric field for any arc by substituting the subtended angle. For a full circle, we see that,
Formula used:
Electric field,
Complete step-by-step solution
We are given an arc of linear charge density
The arc is subtending angle

Now resolving the electric field due to small element of the arc
So we have
We know that electric due to charge dQ is given by,
But,
Substituting (2) in (3),
Now substituting the value of K as
Hence, we find the electric field at the centre of an arc of linear charge density
Note: If the given arc subtended
Similarly, you could find the electric field for any arc by substituting the subtended angle. For a full circle, we see that,
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write a short note on Franklands reaction class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE




