Question

Find the equation of normal to the circle \[{{x}^{2}}+{{y}^{2}}+x+y=0.\] This normal passes through (2, 1).

Answer 1

Hint: To solve the given question, we will use the fact that if there is a normal to a circle, then that normal will always pass through the centre of the circle. So, first, we will find the centre of the given circle by comparing the equation of the given circle with the equation of the standard circle: \[{{x}^{2}}+{{y}^{2}}+2gx+2fy+c=0\] whose centre is (– g, – f). After finding the centre, we will find the equation of the normal by applying two-point form of the line and putting (2, 1) and the coordinates of the centre in that equation.

Complete step by step answer:
A normal is a line which is perpendicular to the curve at a certain point or perpendicular to the tangent of the curve at that point. Now, we will use the fact that if a line is normal to the circle then it will pass through the centre of the circle. Thus, the normal to the circle \[{{x}^{2}}+{{y}^{2}}+x+y=0\] passes through its centre.
Now, we will find the centre of the circle given in the question. Its equation is,
\[{{x}^{2}}+{{y}^{2}}+x+y=0.....\left( i \right)\]
The equation of the standard circle is:
\[{{x}^{2}}+{{y}^{2}}+2gx+2fy=0.....\left( ii \right)\]
Now, we will compare the equations (i) and (ii). Thus, we will get,
\[2g=1\]
\[\Rightarrow g=\dfrac{1}{2}\]
\[2f=1\]
\[\Rightarrow f=\dfrac{1}{2}\]
\[c=0\]
Now, the centre of the standard circle is (– g, – f). So, the centre of the circle: \[{{x}^{2}}+{{y}^{2}}+x+y=0\] is \[\left( \dfrac{-1}{2},\dfrac{-1}{2} \right).\]
Let us draw representing all this information.

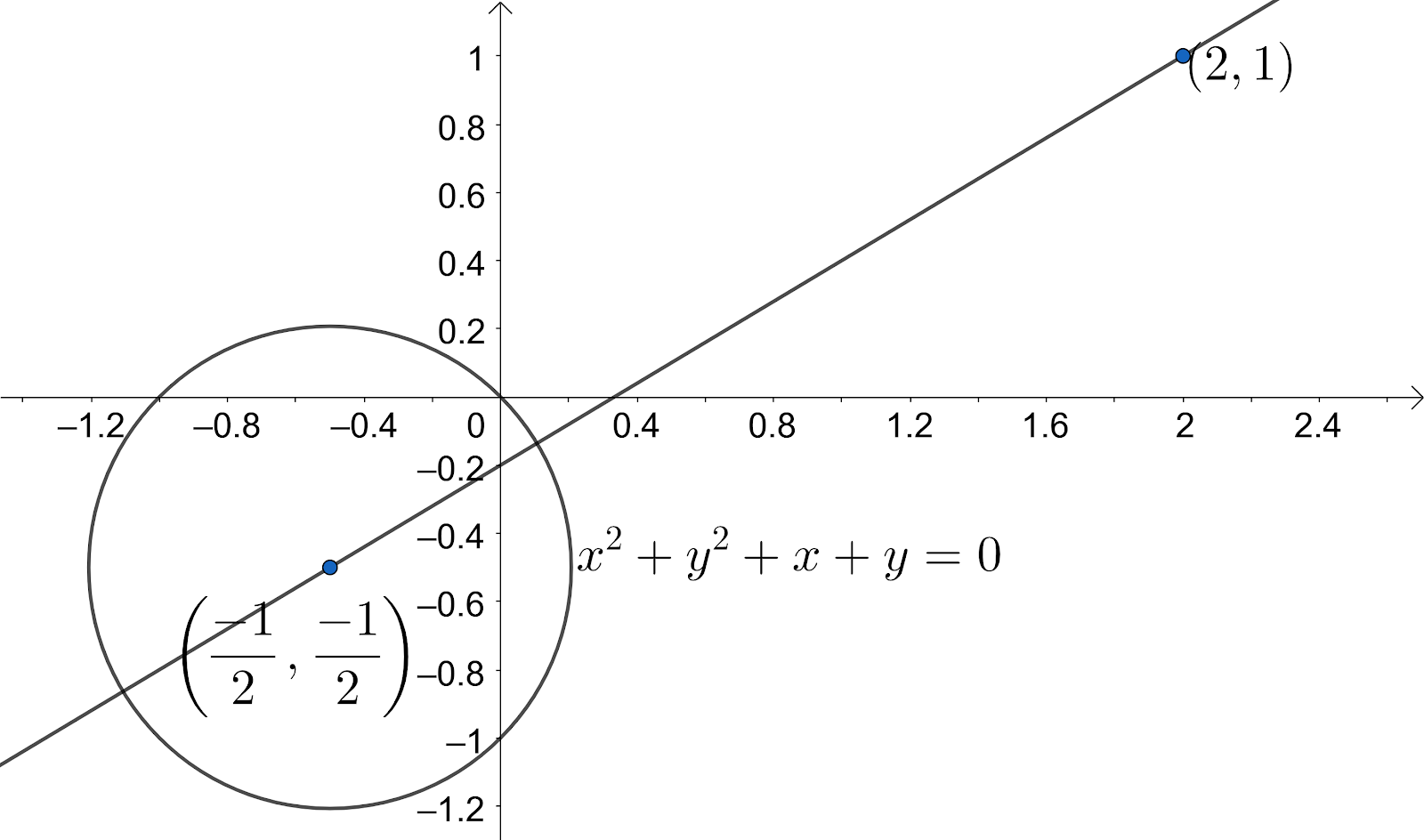
Now, we have got two points on the normal of the line. So, we will use the two point form of the line to get the equation of the normal line. The two point form of the line is
\[y-{{y}_{1}}=\dfrac{{{y}_{2}}-{{y}_{1}}}{{{x}_{2}}-{{x}_{1}}}\left( x-{{x}_{1}} \right)\]
Here, in our case, \[\left( {{x}_{1}},{{y}_{1}} \right)=\left( 2,1 \right)\] and \[\left( {{x}_{2}},{{y}_{2}} \right)=\left( \dfrac{-1}{2},\dfrac{-1}{2} \right).\] Therefore, the normal of the line is
\[y-1=\dfrac{\dfrac{-1}{2}-1}{\dfrac{-1}{2}-2}\left( x-2 \right)\]
\[\Rightarrow y-1=\dfrac{\dfrac{-3}{2}}{\dfrac{-5}{2}}\left( x-2 \right)\]
\[\Rightarrow y-1=\dfrac{3}{5}\left( x-2 \right)\]
\[\Rightarrow 5\left( y-1 \right)=3\left( x-2 \right)\]
\[\Rightarrow 5y-5=3x-6\]
\[\Rightarrow 3x-5y=1\]
Thus, the equation of the normal is 3x – 5y = 1

Note:
 We can use an alternate method to find the equation of the line after finding the two points that lie on that line. Let the equation of the normal line be y = mx + c. Now, we know that this line passes through the point (2, 1). So, we will get,
\[1=m\left( 2 \right)+c\]
\[\Rightarrow 2m+c=1.....\left( i \right)\]
Now this line also passes through \[\left( \dfrac{-1}{2},\dfrac{-1}{2} \right).\] So we will get,
\[\dfrac{-1}{2}=m\left( \dfrac{-1}{2} \right)+c\]
\[\Rightarrow \dfrac{-m}{2}+c=\dfrac{-1}{2}\]
\[\Rightarrow m-2c=1....\left( ii \right)\]
From (i), we have,
\[2m+c=1\]
\[\Rightarrow c=1-2m.....\left( iii \right)\]
Putting this value of c in (ii), we will get,
\[\Rightarrow m-2\left( 1-2m \right)=1\]
\[\Rightarrow m-2+4m=1\]
\[\Rightarrow 5m=3\]
\[\Rightarrow m=\dfrac{3}{5}.....\left( iv \right)\]
Now, we put the value of m in (iii). Thus, we will get,
\[\Rightarrow c=1-2\left( \dfrac{3}{5} \right)\]
\[\Rightarrow c=1-\dfrac{6}{5}\]
\[\Rightarrow c=\dfrac{-1}{5}\]
Now, the equation of the normal line is
\[\Rightarrow y=mx+c\]
\[\Rightarrow y=\dfrac{3x}{5}+\left( \dfrac{-1}{5} \right)\]
\[\Rightarrow 5y=3x-1\]
\[\Rightarrow 3x-5y=1\].