
Find the equation of the hyperbola whose conjugate axis is along the y-axis and having length 3 units and distance between foci is 5.
Answer
529.5k+ views
Hint:In this question start with using the general equation of hyperbola which is
Complete step-by-step answer:
Solution stats here Let the standard equation of hyperbola is
Where (a) and (b) are along the transverse and conjugate axis (i.e. along x-axis and y-axis) and it is half of the length of the transverse axis and conjugate axis.
Now it is given that hyperbola has conjugate axis along y axis and has length 3 units.
Therefore our assumption is right,
And 2b = 3.
Now it is given that the distance between the foci is 5.
Now as we know distance between foci = 2ae, where (e is the eccentricity of hyperbola and always greater than 1.)
Now as we know a, b and e are related as in hyperbola
Now from equation (2) and (3) we have,
Now from equation (1) we have,
Now substitute the values of a and b we have,
Now simplify it we have,
So this is the required equation of hyperbola.
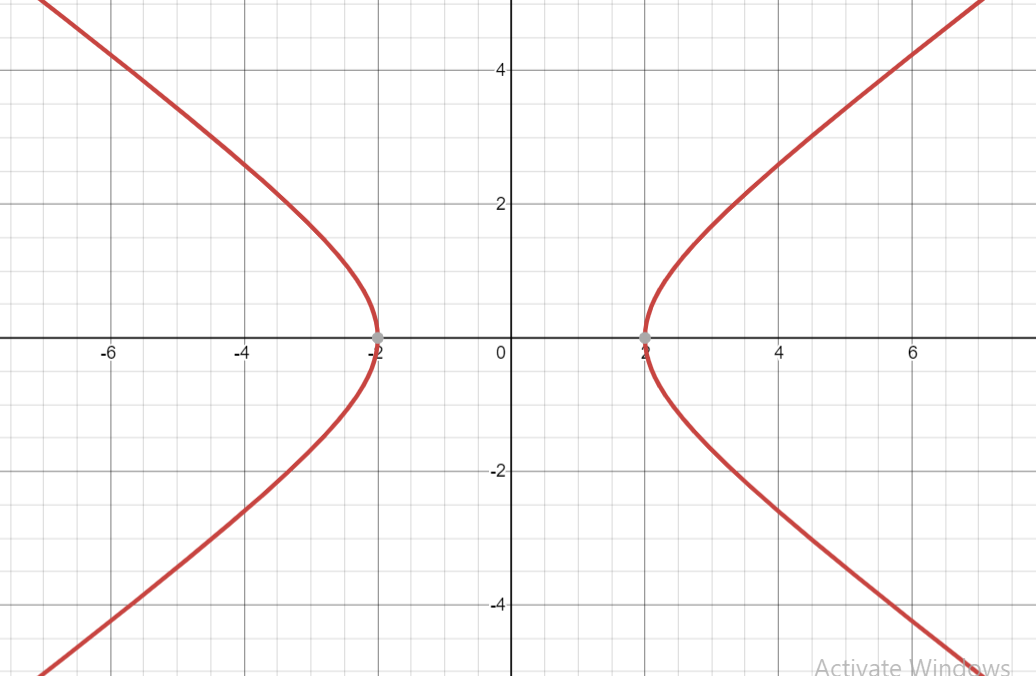
Note – Hyperbola is a symmetrical open curve formed by intersection of a circular cone with a plane at a smaller angle with the axis than the side of the cone. It’s important to see the diagrammatic representation of the equation of the hyperbola obtained as it will help in solving problems of this kind. The graph for hyperbola
Complete step-by-step answer:
Solution stats here Let the standard equation of hyperbola is
Where (a) and (b) are along the transverse and conjugate axis (i.e. along x-axis and y-axis) and it is half of the length of the transverse axis and conjugate axis.
Now it is given that hyperbola has conjugate axis along y axis and has length 3 units.
Therefore our assumption is right,
And 2b = 3.
Now it is given that the distance between the foci is 5.
Now as we know distance between foci = 2ae, where (e is the eccentricity of hyperbola and always greater than 1.)
Now as we know a, b and e are related as in hyperbola
Now from equation (2) and (3) we have,
Now from equation (1) we have,
Now substitute the values of a and b we have,
Now simplify it we have,
So this is the required equation of hyperbola.
Note – Hyperbola is a symmetrical open curve formed by intersection of a circular cone with a plane at a smaller angle with the axis than the side of the cone. It’s important to see the diagrammatic representation of the equation of the hyperbola obtained as it will help in solving problems of this kind. The graph for hyperbola

Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




