
How do you find the focus, vertex, and directrix of
Answer
463.5k+ views
Hint: From the question given, we have been asked to find the focus, vertex and directrix of
Complete step by step answer:
From the question given, we have been given that
We can write the above equation as
We can clearly observe that the given equation from the question is in the form of
If it is so, then its vertex is
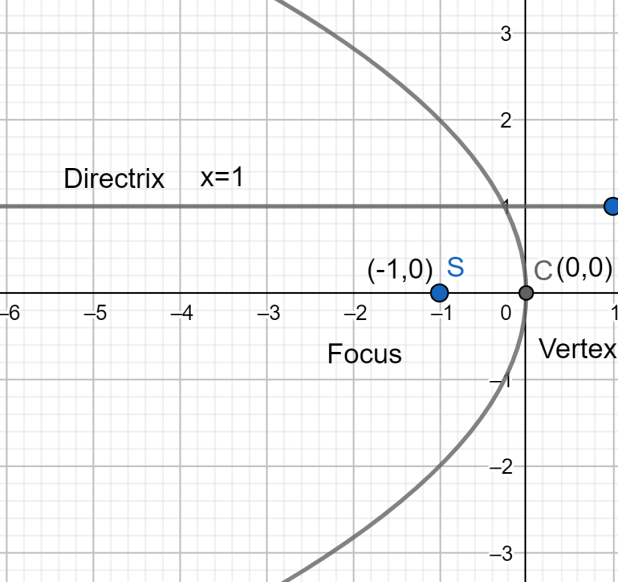
Now, we have to compare the terms and coefficients of both the equations to get the focus, vertex and directrix for the given equation.
By comparing both the equations, we get
Its vertex is
Therefore, we got the focus, vertex and directrix for the given equation.
Note:
We should be very careful while comparing the given equation and general form of the equation. Also, we should be well aware of the concepts of geometry. Also, we should be well known about the terms like focus, vertex and directrix. Also, we should be very careful while writing the focus and directrix for the given equation. Similar to parabola we have curves like hyperbola, ellipse and many more. For hyperbola the general form is
Complete step by step answer:
From the question given, we have been given that
We can write the above equation as
We can clearly observe that the given equation from the question is in the form of
If it is so, then its vertex is

Now, we have to compare the terms and coefficients of both the equations to get the focus, vertex and directrix for the given equation.
By comparing both the equations, we get
Its vertex is
Therefore, we got the focus, vertex and directrix for the given equation.
Note:
We should be very careful while comparing the given equation and general form of the equation. Also, we should be well aware of the concepts of geometry. Also, we should be well known about the terms like focus, vertex and directrix. Also, we should be very careful while writing the focus and directrix for the given equation. Similar to parabola we have curves like hyperbola, ellipse and many more. For hyperbola the general form is
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




