
Find the gravitational force acting on a particle A inside a uniform spherical layer of matter.
Answer
494.4k+ views
Hint: Divide the sphere into thin spherical shells and find the force acting on the particle lying inside the sphere by a thin spherical shell and integrate within the suitable limits.
Complete step by step solution:
Let M be the mass of the uniform spherical layer of matter and m be the mass of the particle lying inside it at a distance of r from the centre. Let the sphere be divided into thin spherical shells of density
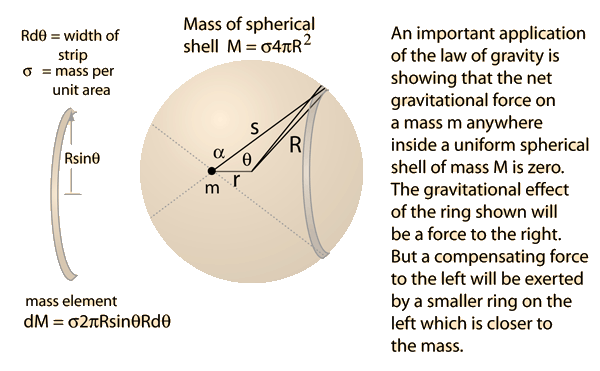
Mass =
The force from the entire spherical shell is given by
In order to find the value of the integral we need to express in terms of. To do so we use cosine formula. Using the cosine law, we have
Differentiating both sides, we get
Now using the cosine formula for the external angle
Now, put the values of equations (2) and (3) in equation (1). Also, for
Now (1) reduces to
Using the area density expression
Thus the algebraic sum of the forces acting on the particle lying inside a sphere is zero.
Note: The gravitational force acting on a particle lying inside a spherical layer of matter is always zero. This is true for all particles lying inside a sphere.
Complete step by step solution:
Let M be the mass of the uniform spherical layer of matter and m be the mass of the particle lying inside it at a distance of r from the centre. Let the sphere be divided into thin spherical shells of density

Mass =
The force from the entire spherical shell is given by
In order to find the value of the integral we need to express in terms of. To do so we use cosine formula. Using the cosine law, we have
Differentiating both sides, we get
Now using the cosine formula for the external angle
Now, put the values of equations (2) and (3) in equation (1). Also, for
Now (1) reduces to
Using the area density expression
Thus the algebraic sum of the forces acting on the particle lying inside a sphere is zero.
Note: The gravitational force acting on a particle lying inside a spherical layer of matter is always zero. This is true for all particles lying inside a sphere.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




