Question

Find the harmonic conjugate of the point R (5,1) with respect to point P (2,10) and Q (6, -2).

Answer 1

Hint: Harmonic conjugate of any point with respect to any two given points by using the concept of external and internal division of line segment. If a point divides another two points in internal ratio, then calculate the coordinates of that point which divide the same two points externally and vice-versa as well. External and internal division of $({{x}_{1}},{{y}_{1}})$ and $({{x}_{2}},{{y}_{2}})$ with ratio of m: n is given as
\[\begin{align}
  & \left( \dfrac{m{{x}_{2}}+n{{x}_{1}}}{m+n},\dfrac{m{{y}_{2}}+n{{y}_{1}}}{m+n} \right)(Internal) \\
 & \left( \dfrac{m{{x}_{2}}-n{{x}_{1}}}{m-n},\dfrac{m{{y}_{2}}-n{{y}_{1}}}{m-n} \right)(External) \\
\end{align}\]

Complete step by step answer:

As we know harmonic conjugate of a point with respect to two points can be given in the following way:
Let us suppose the point \[({{x}_{1}},{{y}_{1}})\]is lying on points \[({{x}_{2}},{{y}_{2}})\] and \[({{x}_{3}},{{y}_{3}})\] such that point \[({{x}_{1}},{{y}_{1}})\] is dividing the given line in the ratio of k:1 internally, then harmonic conjugate of point \[({{x}_{1}},{{y}_{1}})\] with respect to the point $({{x}_{2}},{{y}_{2}})$ and $({{x}_{3}},{{y}_{3}})$ can be calculated by taking the ratio k:1 as an external division i.e. the point which divides the line joining $({{x}_{2}},{{y}_{2}})$ and $({{x}_{3}},{{y}_{3}})$ in ratio of k:1 externally, is known as the harmonic conjugate of the given point $({{x}_{1}},{{y}_{1}})$. And similarly, if $({{x}_{1}},{{y}_{1}})$ divides the points externally in K:1, then we need to calculate the harmonic conjugate by applying the ratio k:1 externally to the given points.
So, we know the sectional formula for internal and external division are given as

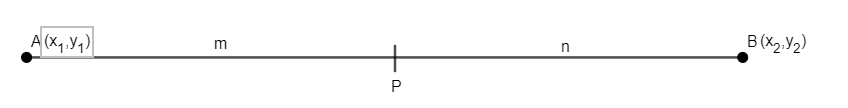
If point P divides the above line by internally, then coordinate of point P is given as
$\left( \dfrac{m{{x}_{2}}+n{{x}_{1}}}{m+n},\dfrac{m{{y}_{2}}+n{{y}_{1}}}{m+n} \right)......(i)$
And if P divides the line by externally, then point P is given as
$\left( \dfrac{m{{x}_{2}}-n{{x}_{1}}}{m-n},\dfrac{m{{y}_{2}}-n{{y}_{1}}}{m-n} \right)......(ii)$
Now coming to the question, as we need to calculate the harmonic conjugate of point R (5, 1) with respect to point P (2, 10) and Q (6, -2). So, suppose point R is dividing line PQ in the ratio k:1.
So, we get

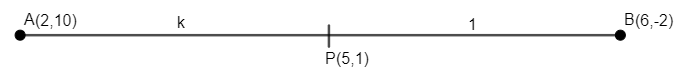
Now, we can get point R with help of equation (i) as
$R=\left( \dfrac{6k+2}{k+1},\dfrac{-2k+10}{k+1} \right).......(iii)$
As, we know the coordinates of R as (5, 1) from the problem. So, we can equate x-coordinate of point R in terms of k from equation (iii) and given in the problem. So, we get
$\begin{align}
  & \dfrac{6k+2}{k+1}=5 \\
 & 6k+2=5k+5 \\
 & k=3 \\
\end{align}$
As we found the ratio 3:1. As the value of k is positive, it means point R will divide the line segment PQ internally. So, harmonic conjugation can be calculated by taking the ratio 3: 1 externally.
So, we can put m = 3 and n = 1 to the expression (ii) to get the harmonic conjugate of the given point. So, let point M is the harmonic conjugate of point R. So, it can be given as
$M=\left( \dfrac{m{{x}_{2}}-n{{x}_{1}}}{m-n},\dfrac{m{{y}_{2}}-n{{y}_{1}}}{m-n} \right)$
Put m = 3, n = 1, $({{x}_{1}},{{y}_{1}})=(2,10)$and $({{x}_{2}},{{y}_{2}})=(6,-2)$
$\begin{align}
  & M=\left( \dfrac{3\times 6-1\times 2}{3-1},\dfrac{3\times (-2)-1\times 10}{3-1} \right) \\
 & M=\left( \dfrac{18-2}{2},\dfrac{-6-10}{2} \right)=(8,-8) \\
 & M=(8,-8) \\
\end{align}$
Hence the harmonic conjugate of the point R (5,1) is (8, -8).

Note: One may suppose the point (5,1) will divide the line segment PQ in the external ratio but value of harmonic conjugate will not change as value of k will changed and hence, we need to use internal division formula for calculating harmonic conjugate
Don’t use the sectional formula as \[\left( \dfrac{m{{x}_{2}}+n{{y}_{2}}}{m+n},\dfrac{m{{x}_{1}}+n{{y}_{1}}}{m+nn} \right)\] ,which is wrong as places of terms \[({{x}_{1}},{{y}_{1}})\]and $({{x}_{2}},{{y}_{2}})$ are changed. So, be clear with the position of $(m,n,{{x}_{1}},{{x}_{2}},{{y}_{1}},{{y}_{2}})$ to get the accurate answer.