
Find the length of the arc in terms of
Answer
530.1k+ views
Hint: First of all convert
Here, we are given a circle of radius 4 cm in which an arc subtends an angle of
First of all, we must know that an arc of a circle is a portion of the circumference of the circle. The length of the arc is simply the length of its portion of circumference. Also, the circumference itself can be considered a full circle arc length.
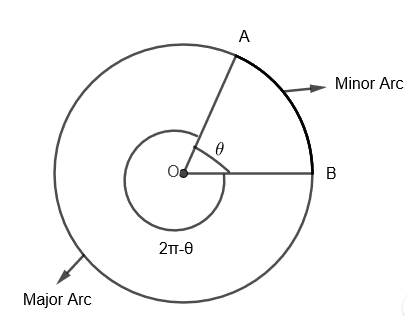
We have two types of arc between any two points in a circle. One is the major arc and other is the minor arc. We know that the total angle at the center of the circle is
Here, as we can see,
Now, we know the circumference of the circle
We know that circumference is also an arc of full circle arc length. Therefore, we can say that the length of the arc made by
By dividing
By multiplying
Now, we have to find the length of the arc which subtends an angle of
We can show it diagrammatically as follows,
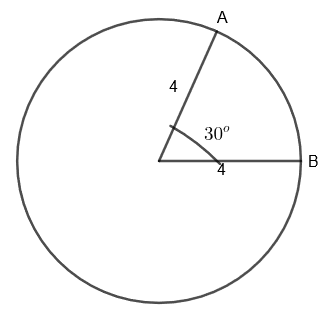
Here, we have to find the length of minor arc AB. As we have to find the length in terms of
We know that, to convert any angle from degree to radians, we must multiply it by
So, we get
By simplifying, we get
Now, to find the length of arc subtended by
We will put
Length of the arc made by
Therefore, we get the length of the arc which subtends an angle of
Note: Students can also find the length of the arc directly by remembering the following formulas.
Length of arc =
Also, students must properly read if the major or minor arc is asked in question and use
Here, we are given a circle of radius 4 cm in which an arc subtends an angle of
First of all, we must know that an arc of a circle is a portion of the circumference of the circle. The length of the arc is simply the length of its portion of circumference. Also, the circumference itself can be considered a full circle arc length.

We have two types of arc between any two points in a circle. One is the major arc and other is the minor arc. We know that the total angle at the center of the circle is
Here, as we can see,
Now, we know the circumference of the circle
We know that circumference is also an arc of full circle arc length. Therefore, we can say that the length of the arc made by
By dividing
By multiplying
Now, we have to find the length of the arc which subtends an angle of
We can show it diagrammatically as follows,

Here, we have to find the length of minor arc AB. As we have to find the length in terms of
We know that, to convert any angle from degree to radians, we must multiply it by
So, we get
By simplifying, we get
Now, to find the length of arc subtended by
We will put
Length of the arc made by
Therefore, we get the length of the arc which subtends an angle of
Note: Students can also find the length of the arc directly by remembering the following formulas.
Length of arc =
Also, students must properly read if the major or minor arc is asked in question and use
Latest Vedantu courses for you
Grade 6 | CBSE | SCHOOL | English
Vedantu 6 Pro Course (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE




