
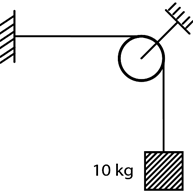
Find the magnitude of force exerted on string by pulley.

Answer
492.6k+ views
Hint: The normal reaction would be balancing the forces of tension from string on left and downward sides. So, it would equal the opposite of the resultant tension forces.
Complete step by step answer:First we will draw the F.B.D of the box and the pulley. As we can see from the question diagram, there would be two forces acting on the box- tension due to string (say T) in the upward direction and weight (say mg) in the downward direction. On the pulley there would be three forces, tension due to string towards left and down , and normal reaction towards string. The tension would be the same as the tension on the box from string because the string is uniform and doesn’t undergo any knot or blockage. Let the tensions be T and normal reactions be N.
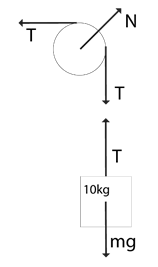
Now, considering equilibrium we can apply the equilibrium condition.
On box,
There are no forces in x-direction.
For y direction,
On pulley, normal reaction can be divided in rectangular components in x-y plane as
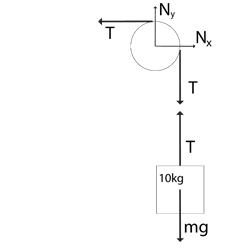
Now balancing the forces in x and y directions, we get
Therefore, normal reaction would be the vector sum of normal reaction components in x and y direction.
Therefore, the correct answer is the pulley exerts a force of
Note:We can also first calculate the total tension forces on the pulley and then equate it to the normal reaction force i.e.
Complete step by step answer:First we will draw the F.B.D of the box and the pulley. As we can see from the question diagram, there would be two forces acting on the box- tension due to string (say T) in the upward direction and weight (say mg) in the downward direction. On the pulley there would be three forces, tension due to string towards left and down , and normal reaction towards string. The tension would be the same as the tension on the box from string because the string is uniform and doesn’t undergo any knot or blockage. Let the tensions be T and normal reactions be N.

Now, considering equilibrium we can apply the equilibrium condition.
On box,
There are no forces in x-direction.
For y direction,
On pulley, normal reaction can be divided in rectangular components in x-y plane as

Now balancing the forces in x and y directions, we get
Therefore, normal reaction would be the vector sum of normal reaction components in x and y direction.
Therefore, the correct answer is the pulley exerts a force of
Note:We can also first calculate the total tension forces on the pulley and then equate it to the normal reaction force i.e.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




