
Find the number of points of non-differentiability of
Answer
492k+ views
Hint: To solve this question we will draw graph of sin x and cos x. Then we will combine the graph and delete the minimum part so that we get the graph of
Now we will check the points where the function is non-differentiable as at that point the curve will not be smooth.
Complete step-by-step solution:
Now let us first understand the concept of differentiability.
A function is called differentiable at a point if its derivative exists at a point.
Now a function is called differentiable if it is differentiable at each point in its domain.
Now let us see the condition for which the function is differentiable.
A function f is said to be differentiable at point c if
Now to check if the limits exist we will check if the left-hand limit is equal to the right-hand limit.
Now let us understand this geometrically.
Now geometrically we know that derivative at a point is nothing but the slope of the curve at that point.
For this derivative to exist we need the curve to be smooth at that point.
Hence if a curve is smooth at a point then we can say it is differentiable
If a curve has sharp point on the curve then the point will be non-differentiable.
Now for example consider
We know that the graph of
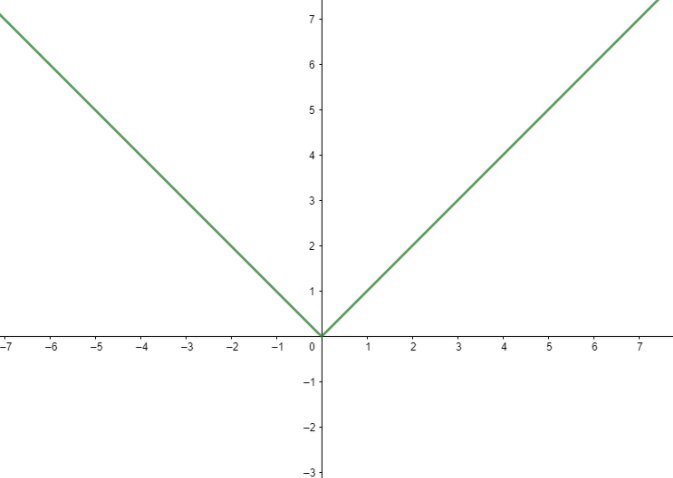
Now we can see from the graph that the graph has a sharp point at
Hence by looking at the graph we can say that the function is not differentiable at
Now consider the given function
Now let us first check the graph of sin x for
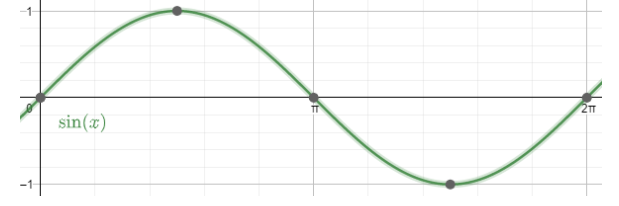
Now let us also check the graph of cos x for
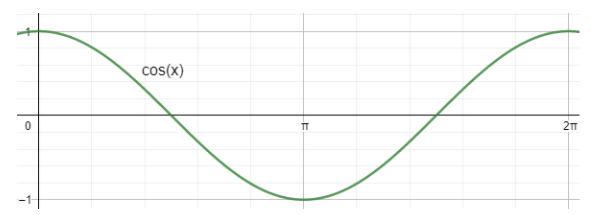
Now we will merge the graph and just consider the maximum part. That means if
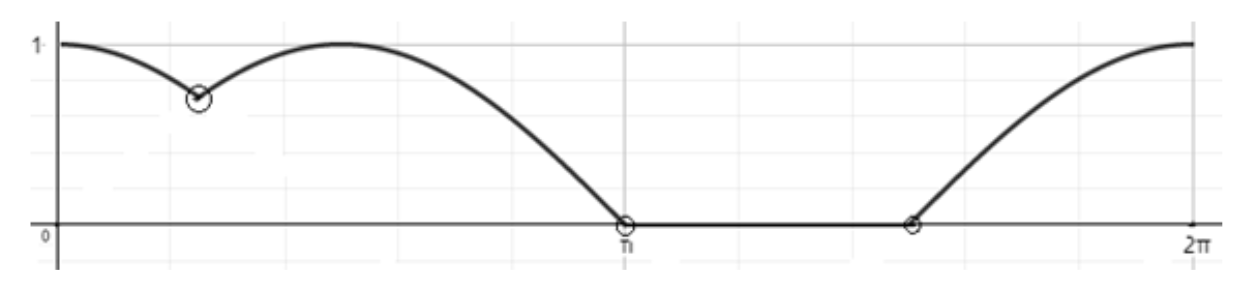
Hence as we can see that the function is sharp at three points and hence is non-differentiable at three points.
Note: Note that whenever the function is defined as a maximum or minimum of two or more functions, we use a geometrical approach to the question as we can easily draw graphs for such functions. Also here we can ask that the function is also not differentiable at point x = 0 and x =
Now we will check the points where the function is non-differentiable as at that point the curve will not be smooth.
Complete step-by-step solution:
Now let us first understand the concept of differentiability.
A function is called differentiable at a point if its derivative exists at a point.
Now a function is called differentiable if it is differentiable at each point in its domain.
Now let us see the condition for which the function is differentiable.
A function f is said to be differentiable at point c if
Now to check if the limits exist we will check if the left-hand limit is equal to the right-hand limit.
Now let us understand this geometrically.
Now geometrically we know that derivative at a point is nothing but the slope of the curve at that point.
For this derivative to exist we need the curve to be smooth at that point.
Hence if a curve is smooth at a point then we can say it is differentiable
If a curve has sharp point on the curve then the point will be non-differentiable.
Now for example consider
We know that the graph of

Now we can see from the graph that the graph has a sharp point at
Hence by looking at the graph we can say that the function is not differentiable at
Now consider the given function
Now let us first check the graph of sin x for

Now let us also check the graph of cos x for

Now we will merge the graph and just consider the maximum part. That means if

Hence as we can see that the function is sharp at three points and hence is non-differentiable at three points.
Note: Note that whenever the function is defined as a maximum or minimum of two or more functions, we use a geometrical approach to the question as we can easily draw graphs for such functions. Also here we can ask that the function is also not differentiable at point x = 0 and x =
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Draw a diagram of a flower and name the parts class 12 biology ICSE




