
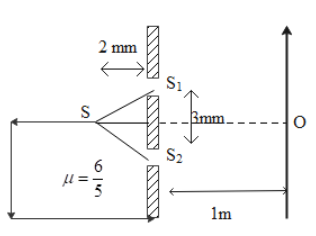
Find the order of the fringe formed at O.

Answer
484.5k+ views
Hint: First calculate the path difference at O. The path difference here is the difference between the
Complete answer:
The path difference is denoted by the letter
Hence,
That is, the distances
Then thickness t is,
Then,
The path length difference must be an integral multiple of the wavelength.
So,
Substituting the value we get,
Additional information:
where, d is the distance between the slits.
D, the distance between the slit and screen.
m and n are the order of interference.
To obtain constructive interference for a double slit, the path length difference must be an integral multiple of the wavelength.
For m= 0,1,-1,2,-2,…… (Constructive interference)
For m=0,1,-1,2,-2,……….(Destructive interference)
Here,
Note:
The value of m and n should not be a decimal or a fractional number. The path difference here is the difference between the
Complete answer:
The path difference is denoted by the letter
Hence,
That is, the distances
Then thickness t is,
Then,
The path length difference must be an integral multiple of the wavelength.
So,
Substituting the value we get,
Additional information:
where, d is the distance between the slits.
D, the distance between the slit and screen.
m and n are the order of interference.
To obtain constructive interference for a double slit, the path length difference must be an integral multiple of the wavelength.
For m= 0,1,-1,2,-2,…… (Constructive interference)
For m=0,1,-1,2,-2,……….(Destructive interference)
Here,
Note:
The value of m and n should not be a decimal or a fractional number. The path difference here is the difference between the
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




