
Find the points of trisection of the line segment joining the points :
(3,-2) and (-3,-4).
Answer
503.4k+ views
Hint : In this question, we will use the concept of section formulae of coordinate geometry. This states that the coordinate of the point which divides the line segment joining the points $({x_1},{y_1})$and $({x_2},{y_2})$ internally in the ratio m:n is given by $\left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right)$. …….(i)
Complete step-by-step solution -
Here , we have given points of the line segment , (3,-2) and (-3,-4)
Comparing this with $({x_1},{y_1})$and $({x_2},{y_2})$, we get
${x_1} = 3,{x_2} = - 3,{y_1} = - 2$ and ${y_2} = - 4$.
We know that trisection means dividing a line segment in three equal parts or dividing a line segment in the ratio 1:2 and 2:1 .
Case 1: when the line segment is divided into m:n as 1:2.
So, m=1 and n=2
Now putting these values in equation (i), we get
$
\Rightarrow \left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right) \\
\Rightarrow \left( {x = \dfrac{{(1)( - 3) + (2)(3)}}{{1 + 2}},y = \dfrac{{(1)( - 4) + (2)( - 2)}}{{1 + 2}}} \right) \\
\Rightarrow \left( {x = \dfrac{{( - 3) + (6)}}{3},y = \dfrac{{( - 4) + ( - 4)}}{3}} \right) \\
\Rightarrow \left( {x = \dfrac{3}{3},y = \dfrac{{ - 8}}{3}} \right) \\
\Rightarrow \left( {x = 1,y = \dfrac{{ - 8}}{3}} \right) \\
$
Hence, the point $\left( {1,\dfrac{{ - 8}}{3}} \right)$ divides the line segment in 1:2.
Case 2: when the line segment is divided into 2:1.
So we have , m=2 and n=1.
Now put these values in equation (i), we get
$
\Rightarrow \left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right) \\
\Rightarrow \left( {x = \dfrac{{(2)( - 3) + (1)(3)}}{{2 + 1}},y = \dfrac{{(2)( - 4) + (1)( - 2)}}{{2 + 1}}} \right) \\
\Rightarrow \left( {x = \dfrac{{( - 6) + (3)}}{3},y = \dfrac{{( - 8) + ( - 2)}}{3}} \right) \\
\Rightarrow \left( {x = \dfrac{{ - 3}}{3},y = \dfrac{{ - 10}}{3}} \right) \\
\Rightarrow \left( {x = - 1,y = \dfrac{{ - 10}}{3}} \right) \\
$
Hence , point $\left( { - 1,\dfrac{{ - 10}}{3}} \right)$ divides the line segment in 2:1 .
Therefore the points of trisection joining the given line segments are $\left( {1,\dfrac{{ - 8}}{3}} \right)$ and $\left( { - 1,\dfrac{{ - 10}}{3}} \right)$ .
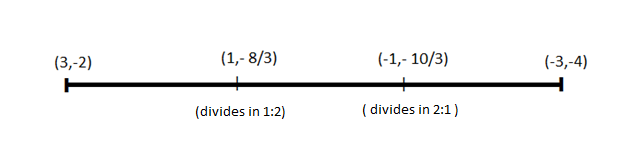
Note : In this type of question we have to remember the concept of the section formulae .first we have to find out the required values and then we will make two case , in one case the ratio m:n is 1:2 and in other case the ratio m:n is 2:1 after that by putting those values in section formulae i.e. $\left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right)$ we will get the required points .
Complete step-by-step solution -
Here , we have given points of the line segment , (3,-2) and (-3,-4)
Comparing this with $({x_1},{y_1})$and $({x_2},{y_2})$, we get
${x_1} = 3,{x_2} = - 3,{y_1} = - 2$ and ${y_2} = - 4$.
We know that trisection means dividing a line segment in three equal parts or dividing a line segment in the ratio 1:2 and 2:1 .
Case 1: when the line segment is divided into m:n as 1:2.
So, m=1 and n=2
Now putting these values in equation (i), we get
$
\Rightarrow \left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right) \\
\Rightarrow \left( {x = \dfrac{{(1)( - 3) + (2)(3)}}{{1 + 2}},y = \dfrac{{(1)( - 4) + (2)( - 2)}}{{1 + 2}}} \right) \\
\Rightarrow \left( {x = \dfrac{{( - 3) + (6)}}{3},y = \dfrac{{( - 4) + ( - 4)}}{3}} \right) \\
\Rightarrow \left( {x = \dfrac{3}{3},y = \dfrac{{ - 8}}{3}} \right) \\
\Rightarrow \left( {x = 1,y = \dfrac{{ - 8}}{3}} \right) \\
$
Hence, the point $\left( {1,\dfrac{{ - 8}}{3}} \right)$ divides the line segment in 1:2.
Case 2: when the line segment is divided into 2:1.
So we have , m=2 and n=1.
Now put these values in equation (i), we get
$
\Rightarrow \left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right) \\
\Rightarrow \left( {x = \dfrac{{(2)( - 3) + (1)(3)}}{{2 + 1}},y = \dfrac{{(2)( - 4) + (1)( - 2)}}{{2 + 1}}} \right) \\
\Rightarrow \left( {x = \dfrac{{( - 6) + (3)}}{3},y = \dfrac{{( - 8) + ( - 2)}}{3}} \right) \\
\Rightarrow \left( {x = \dfrac{{ - 3}}{3},y = \dfrac{{ - 10}}{3}} \right) \\
\Rightarrow \left( {x = - 1,y = \dfrac{{ - 10}}{3}} \right) \\
$
Hence , point $\left( { - 1,\dfrac{{ - 10}}{3}} \right)$ divides the line segment in 2:1 .
Therefore the points of trisection joining the given line segments are $\left( {1,\dfrac{{ - 8}}{3}} \right)$ and $\left( { - 1,\dfrac{{ - 10}}{3}} \right)$ .
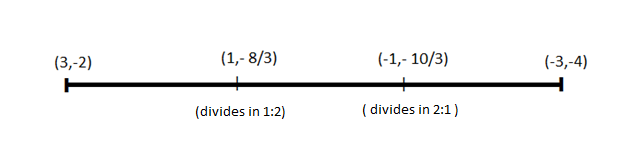
Note : In this type of question we have to remember the concept of the section formulae .first we have to find out the required values and then we will make two case , in one case the ratio m:n is 1:2 and in other case the ratio m:n is 2:1 after that by putting those values in section formulae i.e. $\left( {x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}}} \right)$ we will get the required points .
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

What percentage of the area in India is covered by class 10 social science CBSE

The area of a 6m wide road outside a garden in all class 10 maths CBSE

What is the electric flux through a cube of side 1 class 10 physics CBSE

If one root of x2 x k 0 maybe the square of the other class 10 maths CBSE

The radius and height of a cylinder are in the ratio class 10 maths CBSE

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

What constitutes the central nervous system How are class 10 biology CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE

Explain the Treaty of Vienna of 1815 class 10 social science CBSE




