Question

Find the quadrant, where the point of intersection of the lines \[2x - 3y = - 3, - 12 = - 4x + y\] lies.

Answer 1

Hint: First we have to find the point of intersection of the given 2 lines. Next we must check the Quadrant in which the point lies.

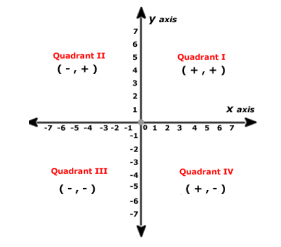
Coordinate axes divide the Cartesian plane in 4 quadrants.

Complete step-by-step answer:
Given: Two equations of line
\[2x - 3y = - 3\] ........ (I)
\[ - 4x + y = - 12\] ........ (II)
Now, by substitution method we solving these equations to get value of \[x,y\]
From equation (II) \[y = - 12 + 4x\] ……. (III)
Put this value in equation (I)
\[2x - 3( - 12 + 4x) = - 3\]
\[2x + 36 - 12x = - 3\]
\[36 - 10x = - 3\]
\[ - 10x = - 3 - 36\]
\[ - 10x = - 39\]
\[x = 3.9\]
Which is positive so, lies in quadrant 1
Now for find the value of by using \[x = 3.9\] in equation (III)
\[y = - 12 + 4(3.90)\]
\[y = - 12 + 15.6\]
\[y = 3.6\]
Which are positive lies in Ist quadrant
Hence, \[x = + 3.9\] & \[y = + 3.6\]
\[(x,y) = ( + 3.9, + 3.6)\] [point of intersection]

Quadrant 1 \[( + , + )\] is the correct answer.

Note: Quadrant: When the axis of two dimensional cartesian systems divide the plane into four infinite regions, then that is called quadrants, each bounded by two half axes. The coordinate plane is divided into four quadrants by horizontal number of lines (the axis) and a vertical line (the axis) that intersect at a point called the origin. When Two or more lines cross each other in a plane they are called intersecting lines. The intersecting lines share a common point, which exists on all the intersecting lines and is called the point of intersection.
There are four quadrant I, II, III, IV for every quadrant sign are fixed which are for 1st it is \[( + , + )\] for 2nd \[( - , + )\], 3rd \[( - , - )\], 4th \[( + , - )\].