
How do you find the range from the undefined graph of
Answer
452.4k+ views
1 likes
Hint: The range of a function is the set of all the values of the dependent variable y which are obtained after substituting all the values of the independent variable x from the domain of the function. Therefore, the range of the given function
Complete step by step solution:
The function given in the question is
We can put
Now, we know the algebraic identity given by
Now, we know that the logarithm function is not defined for the argument of zero. Therefore, the point at which the graph of the given function will not be defined is given by
Therefore, the graph of the given function will not be defined at
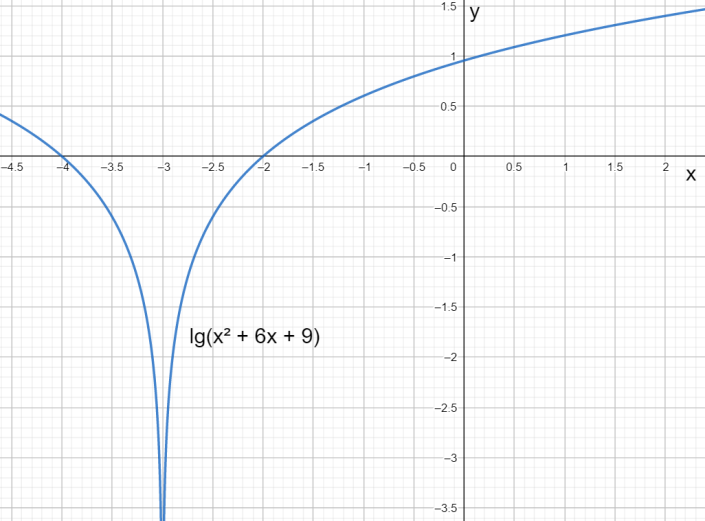
From the above graph we can see that the graph of the given function covers the whole y-axis. Therefore, we can say that the range of the function is
Hence, we have determined the range from the undefined graph of the given function.
Note: We can also determine the range without using the graph by just analyzing the given function. Since the argument to the logarithm function is a polynomial, which takes all the real values, the range of the given function must be the same as that of the function
Complete step by step solution:
The function given in the question is
We can put
Now, we know the algebraic identity given by
Now, we know that the logarithm function is not defined for the argument of zero. Therefore, the point at which the graph of the given function will not be defined is given by
Therefore, the graph of the given function will not be defined at

From the above graph we can see that the graph of the given function covers the whole y-axis. Therefore, we can say that the range of the function is
Hence, we have determined the range from the undefined graph of the given function.
Note: We can also determine the range without using the graph by just analyzing the given function. Since the argument to the logarithm function is a polynomial, which takes all the real values, the range of the given function must be the same as that of the function
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

What are the major means of transport Explain each class 12 social science CBSE




