Question

Find the ratio in which the line $ x - 3y = 0 $ divides the line segment joining the points $ ( - 2, - 5)\,\,and\,\,\left( {6,3} \right) $ . Find the coordinate of the point of intersection.

Answer 1

Hint: To find ratio we first let ratio be $ k:1 $ and then using section formulas to find coordinate of point and then using coordinate in given line as point also lie on line and then solving an equation formed to get value of k or required ratio in which line divides given line segment.
Formulas used: Section formula: $ x = \dfrac{{m{x_2} + n{x_1}}}{{m + n}},\,\,y = \dfrac{{m{y_2} + n{y_1}}}{{m + n}} $

Complete step-by-step answer:
Points on the line segment are $ ( - 2, - 5)\,\,and\,\,\left( {6,3} \right) $ .
To find the required ratio.
We first let the ratio be $ k:1 $ . In which line segment $ x - 3y = 0 $ divides points $ ( - 2, - 5)\,\,and\,\,\left( {6,3} \right) $ .

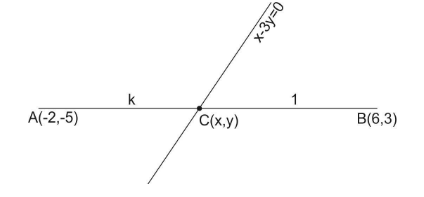
Then by using section formula coordinate of point C will be given as:
 $
  x = \dfrac{{6(k) + 1( - 2)}}{{k + 1}},\,\,y = \dfrac{{3(k) + 1( - 5)}}{{k + 1}} \\
  x = \dfrac{{6k - 2}}{{k + 1}},\,\,y = \dfrac{{3k - 5}}{{k + 1}} \\
  $
Therefore, coordinate of the point $ C\left( {\dfrac{{6k - 2}}{{k + 1}},\dfrac{{3k - 5}}{{k + 1}}} \right) $
Also, point $ C\left( {\dfrac{{6k - 2}}{{k + 1}},\dfrac{{3k - 5}}{{k + 1}}} \right) $ line on the line $ x - 3y = 0 $ . Therefore, point $ C\left( {\dfrac{{6k - 2}}{{k + 1}},\dfrac{{3k - 5}}{{k + 1}}} \right) $ will satisfy given line.
Substituting values in given line:
\[
  \dfrac{{6k - 2}}{{k + 1}} - 3\left( {\dfrac{{3k - 5}}{{k + 1}}} \right) = 0 \\
   \Rightarrow \dfrac{{6k - 2 - 9k + 15}}{{k + 1}} = 0 \\
   \Rightarrow \dfrac{{ - 3k + 13}}{{k + 1}} \;
   \Rightarrow - 3k + 13 = 0 \\
   \Rightarrow - 3k = - 13 \\
   \Rightarrow k = \dfrac{{13}}{3} \;
 \]
Therefore, line $ x - 3y = 0 $ divides line segment joining points $ ( - 2, - 5)\,\,and\,\,\left( {6,3} \right) $ is $ 13:3 $ .

To find the point of intersection or intercept we substitute the value of ratio obtained above in section formula. We have,
 $ C\left( {\dfrac{{6k - 2}}{{k + 1}},\dfrac{{3k - 5}}{{k + 1}}} \right) $
 $
   \Rightarrow C\left( {\dfrac{{6\left( {\dfrac{{13}}{3}} \right) - 2}}{{\dfrac{{13}}{3} + 1}},\dfrac{{3\left( {\dfrac{{13}}{3}} \right) - 5}}{{\dfrac{{13}}{3} + 1}}} \right) \\
   \Rightarrow C\left( {\dfrac{{\dfrac{{78}}{3} - 2}}{{\dfrac{{16}}{3}}},\dfrac{{\dfrac{{39}}{3} - 5}}{{\dfrac{{16}}{3}}}} \right) \\
   \Rightarrow C\left( {\dfrac{{\dfrac{{78 - 6}}{3}}}{{\dfrac{{16}}{3}}},\dfrac{{\dfrac{{39 - 15}}{3}}}{{\dfrac{{16}}{3}}}} \right) \\
   \Rightarrow C\left( {\dfrac{{\dfrac{{72}}{3}}}{{\dfrac{{16}}{3}}},\dfrac{{\dfrac{{24}}{3}}}{{\dfrac{{16}}{3}}}} \right) \\
   \Rightarrow C\left( {\dfrac{{72}}{{16}},\dfrac{{24}}{{16}}} \right) \\
   \Rightarrow C\left( {\dfrac{9}{2},\dfrac{3}{2}} \right) \;
  $
Therefore, the coordinate of the point of intersection is $ \left( {\dfrac{9}{2},\dfrac{3}{2}} \right) $ .
So, the correct answer is “$ \left( {\dfrac{9}{2},\dfrac{3}{2}} \right) $”.

Note: In this type of problems in which ratio is required. If on solving we get a ratio as positive then point will lie in between the given line segment and if ratio obtained is negative then point will lie outside the given line segment.