
Find the shortest and the longest distance from the point (2,-7) to the circle
Answer
522.6k+ views
Hint: In this question it is given that we have to find the shortest and the longest distance from the point (2,-7) to the circle
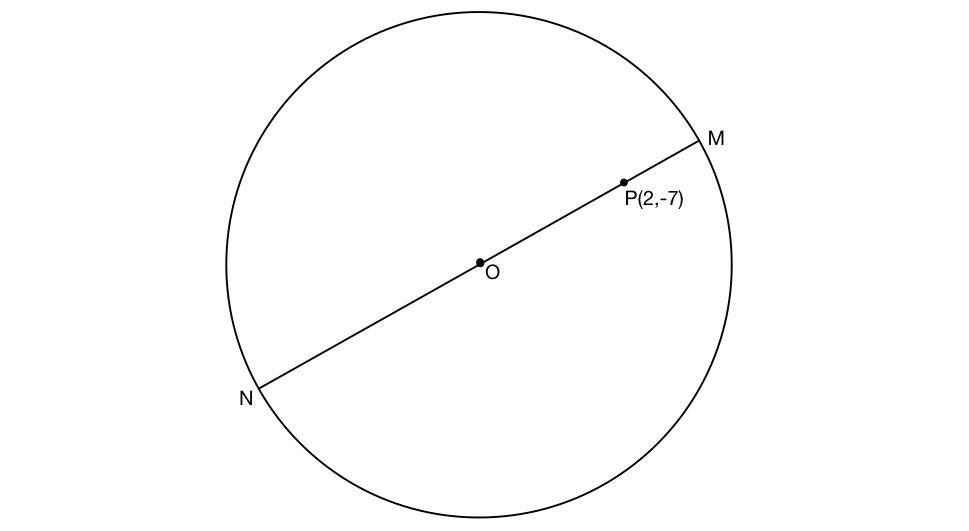
So we have to find the coordinate of the centre O and the radius of the circle i.e, OM=ON=radius. And after that we can easily find the shortest distance PM=OM-OP and longest distance PN=MN-PM.
Complete step-by-step answer:
So before moving into the coordinates first of all we have to determine that the point P(2,-7) lies in which side of circle,
So putting the value x=2, y=-7 in left hand side of the equation we get,
=
=4+49-28+70-151 = -56 <0.
And as we know that if any point (a,b) lies inside the circle then we can write,
So the given point P(2,-7) lies inside the circle.
Now as we know that if any equation of circle is in the form of
So comparing the above equation with the given equation
r=OM=ON=
=
For finding distance we need to know the distance formula i.e, the distance between the points (a,b) to (c,d) is
So. by the above formula we can find the distance between centre O(7,5) and P(2,-7)
OP=
=
=
=
Then the shortest distance from the point P(2,-7) to the circle,
PM=OM-OP=15-13=2 units.
And the longest distance,
PN=MN-PM=(OM+ON)-PM =(15+15)-2=28 units.
Note: To solve this type of question you need to keep in mind that every point inside the circle must lie on one of its diameters. And then the point divides the diameter into two parts so the length of one part must be the longest one and the other one is the shortest.

So we have to find the coordinate of the centre O and the radius of the circle i.e, OM=ON=radius. And after that we can easily find the shortest distance PM=OM-OP and longest distance PN=MN-PM.
Complete step-by-step answer:
So before moving into the coordinates first of all we have to determine that the point P(2,-7) lies in which side of circle,
So putting the value x=2, y=-7 in left hand side of the equation we get,
=
=4+49-28+70-151 = -56 <0.
And as we know that if any point (a,b) lies inside the circle then we can write,
So the given point P(2,-7) lies inside the circle.
Now as we know that if any equation of circle is in the form of
So comparing the above equation with the given equation
r=OM=ON=
=
For finding distance we need to know the distance formula i.e, the distance between the points (a,b) to (c,d) is
So. by the above formula we can find the distance between centre O(7,5) and P(2,-7)
OP=
=
=
=
Then the shortest distance from the point P(2,-7) to the circle,
PM=OM-OP=15-13=2 units.
And the longest distance,
PN=MN-PM=(OM+ON)-PM =(15+15)-2=28 units.
Note: To solve this type of question you need to keep in mind that every point inside the circle must lie on one of its diameters. And then the point divides the diameter into two parts so the length of one part must be the longest one and the other one is the shortest.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




