
Find the square meters, the area of rectangle of length
Answer
511.2k+ views
Hint: Find a formula for area of rectangle. Now substitute the values of variables you need to solve the equation. By this you will get the value of the area of the rectangle. This area is the required result in the question.
Complete step-by-step answer:
Rectangle: In Euclidean plane geometry, a rectangle is a quadrilateral with 4 right angles. It can also be defined as an equiangular quadrilateral, since equiangular means that all of its angles are equal. It can also be defined as a parallelogram containing a right angle. It is a two-dimensional shape.
Besides the above definition we can also define the rectangle as a combination of strips of breadth db upto b each of length l.
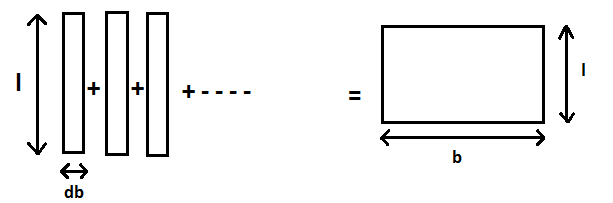
As you can see if we integrate the area of the strip from
As l does not depend on b we can bring it out.
By basic properties of integration, we know the relation as:
By substituting this, we can write the equation as:
By substituting the limits into function, we get the equation:
By simplifying the above equation, we can write it as:
Given conditions in the question, can be written as follows:
The length of rectangle is given by the equation:
The breadth of rectangle is given by the equation:
Area of rectangle can be found by substituting these:
We need to convert 12m into cms.
By basic knowledge of measurement we know the relation
By multiplying with 125 on both sides, we get the equation
By substituting this into equation (1) we can write it as:
By simplifying above we can write it as follows:
The value of area in square meters is
Note: Be careful while integrating generally students confuse and write l(dl) where they get a quadratic but that is for square here we write l(db) which leads to polynomial lb. Alternatively you can convert 12m into cm and find the area in
Complete step-by-step answer:
Rectangle: In Euclidean plane geometry, a rectangle is a quadrilateral with 4 right angles. It can also be defined as an equiangular quadrilateral, since equiangular means that all of its angles are equal. It can also be defined as a parallelogram containing a right angle. It is a two-dimensional shape.
Besides the above definition we can also define the rectangle as a combination of strips of breadth db upto b each of length l.
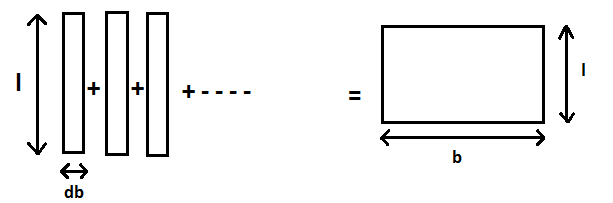
As you can see if we integrate the area of the strip from
As l does not depend on b we can bring it out.
By basic properties of integration, we know the relation as:
By substituting this, we can write the equation as:
By substituting the limits into function, we get the equation:
By simplifying the above equation, we can write it as:
Given conditions in the question, can be written as follows:
The length of rectangle is given by the equation:
The breadth of rectangle is given by the equation:
Area of rectangle can be found by substituting these:
We need to convert 12m into cms.
By basic knowledge of measurement we know the relation
By multiplying with 125 on both sides, we get the equation
By substituting this into equation (1) we can write it as:
By simplifying above we can write it as follows:
The value of area in square meters is
Note: Be careful while integrating generally students confuse and write l(dl) where they get a quadratic but that is for square here we write l(db) which leads to polynomial lb. Alternatively you can convert 12m into cm and find the area in
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




