
Find the value of
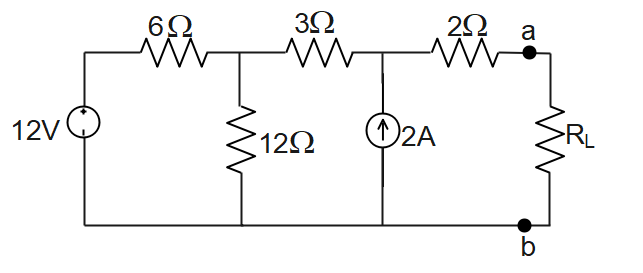

Answer
432.3k+ views
2 likes
Hint: Maximum power transfer theorem is particularly useful for analyzing communication networks because in communication engineering it is usually desirable to deliver maximum power to a load. Electronic amplifiers in radio and television receivers are so designed to do this as it does not matter that an equal amount of power is lost in the process.
Complete step-by-step solution:
Maximum power transfer theorem is stated as follows:
A resistive load, served through a resistive network, will abstract maximum power when the load resistance value is the same as the resistance “viewed by the load as it looks back into the network “.This is called the output resistance of the network or the resistance presented to the output terminals by the network. It is the resistance we called
To find
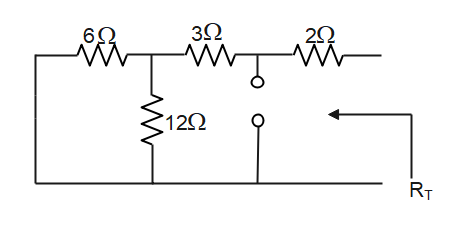
To find
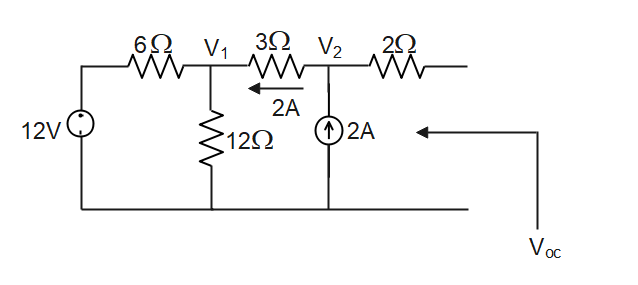
Apply KCL to the circuit
Maximum power transfer will happen when
From maximum power transfer theorem
After substituting
The maximum power is equal to 17.28watt.
Note: Students similarly for a current source the power transferred will be maximum when load conductance is equal to source conductance and maximum power transferred is
Complete step-by-step solution:
Maximum power transfer theorem is stated as follows:
A resistive load, served through a resistive network, will abstract maximum power when the load resistance value is the same as the resistance “viewed by the load as it looks back into the network “.This is called the output resistance of the network or the resistance presented to the output terminals by the network. It is the resistance we called
To find

To find

Apply KCL to the circuit
Maximum power transfer will happen when
From maximum power transfer theorem
After substituting
The maximum power is equal to 17.28watt.
Note: Students similarly for a current source the power transferred will be maximum when load conductance is equal to source conductance and maximum power transferred is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




