Question

Find the value of $\tan {300}^{\circ }$

Answer 1

Hint:We are given a trigonometric function of tangent with an angle of $300$ degrees and we have to find its value. For this we use the Cartesian plane for trigonometric functions’ sine i.e. positive or negative and use the standard table of trigonometric functions value at particular angles.

Complete solution step by step:
Firstly we write down the expression of which we are supposed to find the value
$\tan {300}^{\circ }$
We are given a tangent function whose argument is provided as 300 degrees now we have to see that in which quadrant it lies so that we can get which sign it will take after converting it into standard degree angles whose values are known to us for all six functions. We understand this by the below simplification and given figure (a) i.e.
$\tan {300}^{\circ }=\tan \left({360}^{\circ }-{60}^{\circ }\right)$
We can see that it lies in the fourth quadrant. Now we look at the figure and conclude which sign it will take with it after conversion i.e.

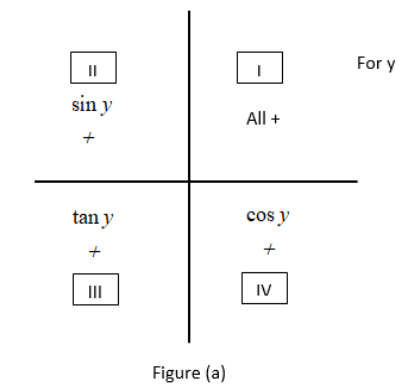
We can clearly see that tangent is positive in third quadrant and first quadrant and it will be negative in the fourth quadrant so we have
$\tan {300}^{\circ }=\tan \left({360}^{\circ }-{60}^{\circ }\right)=-\tan {60}^{\circ }$
We have simplified the value of tangent into standard angle value. Now looking this value up in the below table

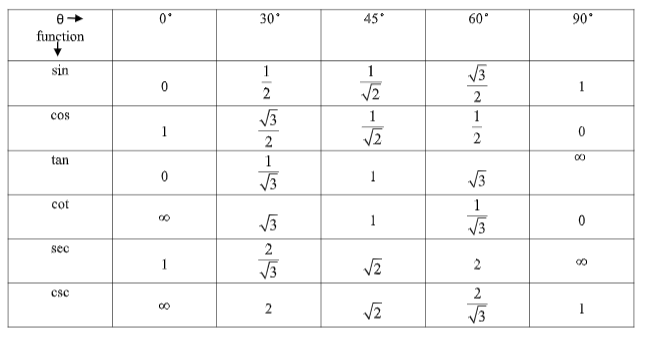
We have
$-\tan {60}^{\circ }=-\sqrt{3}$
This is the final outcome of the given tangent function.

Note: Wherever possible conversion of trigonometric functions should be done with respect to either ${180}^{\circ }$ or$0^{\circ },{360}^{\circ }$ for simpler calculations. Because this conversion does not lead to conversion of function such as sine converts into cosine which happens while doing so with ${90}^{\circ }\text{or}{270}^{\circ }$.