
Find the value of the given trigonometric ratio,
Answer
525.3k+ views
Hint: Use the formula of
Complete step-by-step answer:
We know;
The other commonly used trigonometric values include:
Also, we have, the formula:
So, in the above formula substituting
Putting the value of
On cross-multiplication, we get;
So, the equation we get is a quadratic equation, and one of the roots of this quadratic equation would be the value of
We know, for a quadratic equation of the form
Applying the formula to our quadratic equation, we have;
We know,
According to the graph of
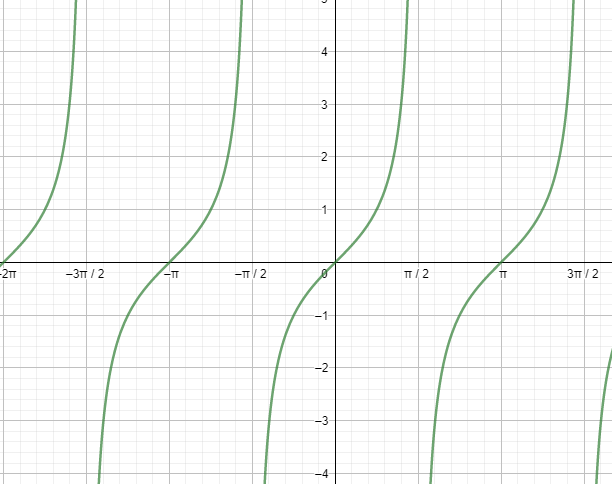
Therefore,
Hence, the value of
Note: Other useful formulas include:
And you are free to use any formula, just substitute the angles according to the need to get the desired values.
We can also find the value of
On Substituting A and B in the above formula, we get;
The equation becomes:
Point to remember: whenever you try to find the value of
Complete step-by-step answer:
We know;
The other commonly used trigonometric values include:
Also, we have, the formula:
So, in the above formula substituting
Putting the value of
On cross-multiplication, we get;
So, the equation we get is a quadratic equation, and one of the roots of this quadratic equation would be the value of
We know, for a quadratic equation of the form
Applying the formula to our quadratic equation, we have;
We know,
According to the graph of

Therefore,
Hence, the value of
Note: Other useful formulas include:
And you are free to use any formula, just substitute the angles according to the need to get the desired values.
We can also find the value of
On Substituting A and B in the above formula, we get;
The equation becomes:
Point to remember: whenever you try to find the value of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




