Question

Find the value of \[x\] in the following figure.

A.\[35^\circ \]
B.\[45^\circ \]
C.\[55^\circ \]
D.None of these

Answer 1

Hint: First we will use the angle sum property that the sum of angles on one side of a straight line is \[180^\circ \]. Then we will add the three angles and take the sum equal to \[180^\circ \] find the required value.

Complete step-by-step answer:
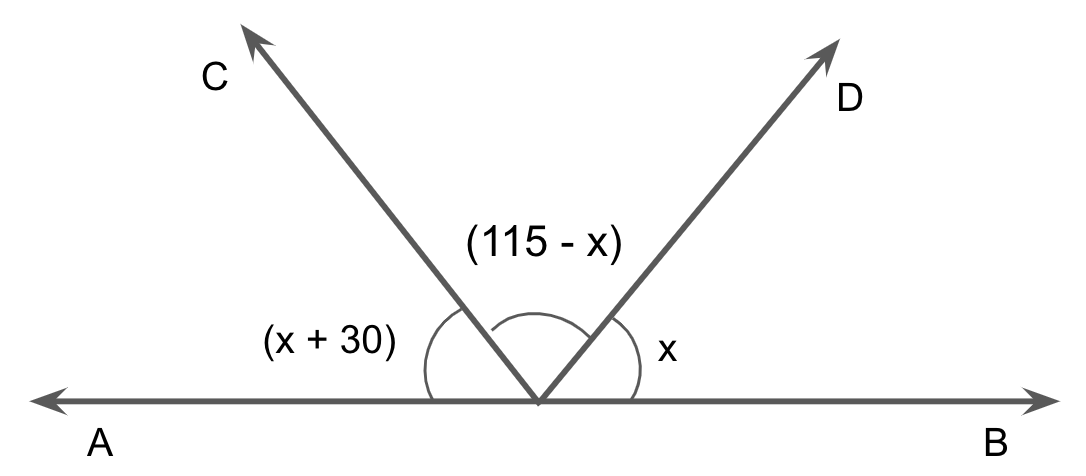
We are given that the angles are \[\left( {x + 30} \right)^\circ \], \[\left( {115 - x} \right)^\circ \] and \[x^\circ \].
We know that the sum of angles on one side of a straight line is \[180^\circ \].
Adding the above three angles, we get
\[
   \Rightarrow \left( {x + 30} \right)^\circ + \left( {115 - x} \right)^\circ + x^\circ \\
   \Rightarrow x^\circ + 30^\circ + 115^\circ - x^\circ + x^\circ \\
   \Rightarrow x^\circ + 145^\circ \\
 \]
Since we know the above sum is equal to \[145^\circ \], we have
\[
   \Rightarrow x^\circ + 145^\circ = 180^\circ \\
   \Rightarrow x^\circ + 145^\circ - 145^\circ = 180^\circ - 145^\circ \\
   \Rightarrow x^\circ = 35^\circ \\
 \]
Thus, the value of \[x\] is 35 degrees.


Note: In solving these types of questions, students need to know the basic properties of angles on a straight line. The possibility of error in this question can be that you assume the sum is equal to 90 degrees, which is wrong. Also, avoid calculation mistakes.