
What is the flux over a curved surface of the hemisphere?

Answer
430.5k+ views
Hint:The rate of flow of electric field lines through a given area is known as electric flux
Complete step by step answer:
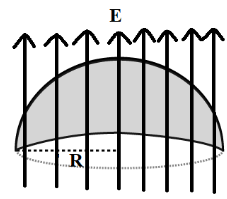
The electric flux over a curved surface area of the hemisphere can be represented as shown in the figure below, let
The electric flux
Where
The angle between the electric field
We know that, the area of circle is
Hence, the flux over a curved surface of the hemisphere is
Note: We need to consider the area of the circle not the area of the hemisphere because the electric field lines pass through the base of the hemisphere and the base of the hemisphere is a circle. Negative sign indicates that the direction of electric field lines and area vectors is opposite.
Complete step by step answer:
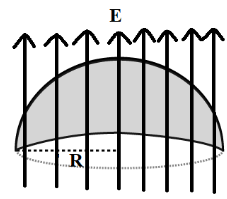
The electric flux over a curved surface area of the hemisphere can be represented as shown in the figure below, let

The electric flux
Where
The angle between the electric field
We know that, the area of circle is
Hence, the flux over a curved surface of the hemisphere is
Note: We need to consider the area of the circle not the area of the hemisphere because the electric field lines pass through the base of the hemisphere and the base of the hemisphere is a circle. Negative sign indicates that the direction of electric field lines and area vectors is opposite.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
What is the difference between resemblance and sem class 12 social science CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

Draw ray diagrams each showing i myopic eye and ii class 12 physics CBSE

Why do the transition elements have higher enthalpies class 12 chemistry CBSE

What are the advantages of parallel combination over class 12 physics CBSE

What are the causes of overloading class 12 physics CBSE




