Question

What is the flux through a cube of side ‘a’ if a point charge q is at one of its corners ?
A) \[\dfrac{q}{{{\varepsilon _\circ }}}\]
B) \[\dfrac{q}{{2{\varepsilon _\circ }}}\]
C) \[\dfrac{{2q}}{{{\varepsilon _\circ }}}\]
D) \[\dfrac{q}{{8{\varepsilon _\circ }}}\]

Answer 1

Hint: Electric flux is the rate of flow of the electric field through a given area. According to gauss law, the electric flux through a closed surface is equal to \[\dfrac{q}{{{\varepsilon _\circ }}}\], if q is the charge enclosed in it.
In case if a charge is placed at one of the corners of the cube then the amount of charge enclosed in it is eighth part of the charge so the flux is also the eighth part of \[\dfrac{q}{{{\varepsilon _\circ }}}\].

Formula used:
Gauss law: According to gauss law :- If a charge “q ” is enclosed in a closed surface then the net flux emerging out of the closed surface is \[\dfrac{q}{{{\varepsilon _\circ }}}\]. Gauss law is only applicable for closed bodies.

Complete step by step solution:
According to gauss law, the electric flux through a closed surface is equal to \[\dfrac{q}{{{\varepsilon _\circ }}}\], if q is the charge enclosed in it.

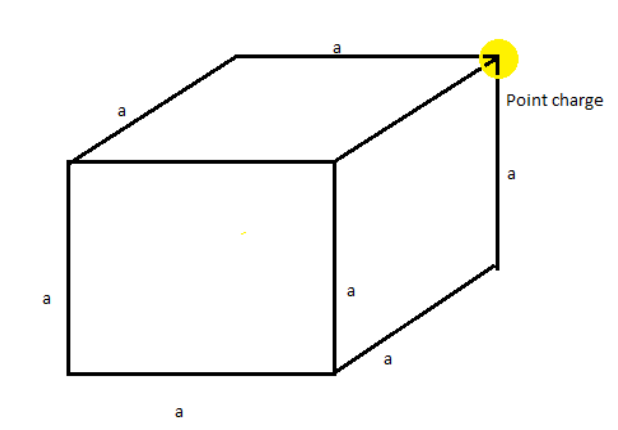
If the charge ‘q ’is placed at one of the corners of the cube, it will be divided into 8 such cubes. Therefore, electric flux through the one cube is the eighth part of \[\dfrac{q}{{{\varepsilon _\circ }}}\].
So electric flux (\[\varphi \]) is equal to \[\dfrac{q}{{8{\varepsilon _\circ }}}\].
Hence option (D) is the correct answer.
Gauss law is one of the four Maxwell’s equations which form the basis of classical electrodynamics.

Note: Electric flux has SI units of volt metres ( V m).
Electric flux is the rate of flow of electric field through a given area. Electric flux is proportional to the number of electric field lines going through a virtual surface. Gauss law can be used to derive the coulomb’s law and vice-versa.