Question

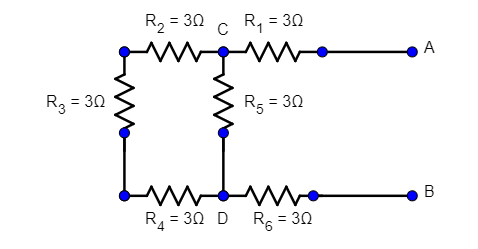
For the combination of resistors shown in the figure, find the equivalent resistance between (a) $C$ and $D$ (b) $A$ and $B$.

Answer 1

Hint: This problem can be solved by using the formulas for equivalent resistances for series and parallel combinations of resistances. For finding the equivalent resistance between two terminals, we have to find the equivalent resistance in all branches of the circuit between the two terminals.

Formula used:
${{R}_{equivalent,series}}=\sum\limits_{i=1}^{k}{{{R}_{i}}}$
$\dfrac{1}{{{R}_{equivalent,parallel}}}=\sum\limits_{i=1}^{k}{\dfrac{1}{{{R}_{i}}}}$

Complete step-by-step answer:
We will have to solve the problem by using the formulas for the equivalent resistances for series and parallel combinations for all the branches between the two given terminals.
The equivalent resistance ${{R}_{equivalent,series}}$ of $k$ resistances in series of individual resistances ${{R}_{i}}\left( i\in \left[ 1,k \right] \right)$ is given by
${{R}_{equivalent,series}}=\sum\limits_{i=1}^{k}{{{R}_{i}}}$ --(1)
The equivalent resistance ${{R}_{equivalent,parallel}}$ of $k$ resistances in parallel of individual resistances ${{R}_{i}}\left( i\in \left[ 1,k \right] \right)$ is given by
$\dfrac{1}{{{R}_{equivalent,parallel}}}=\sum\limits_{i=1}^{k}{\dfrac{1}{{{R}_{i}}}}$ --(2)
Here ${{R}_{1}}={{R}_{2}}={{R}_{3}}={{R}_{4}}={{R}_{5}}={{R}_{6}}=3\Omega $

(a) Equivalent resistance between $C$ and $D$ -
The branches connecting $C$ and $D$ are the two branches, one containing ${{R}_{5}}$ and the other containing resistors ${{R}_{2}},{{R}_{3}},{{R}_{4}}$.
The other branch that is the one having nodes $A$ and $B$ is a broken circuit. Hence, ${{R}_{1}},{{R}_{6}}$ are not considered for the equivalent resistance.
Therefore, the equivalent resistance is the parallel combination of the two branches, one containing ${{R}_{5}}$ and the other containing resistors ${{R}_{2}},{{R}_{3}},{{R}_{4}}$.
$\therefore {{R}_{CD}}={{R}_{2,3,4}}||{{R}_{5}}$ --(3)
Now, ${{R}_{2}},{{R}_{3}},{{R}_{4}}$ are in series. Therefore using (1) their equivalent will be
${{R}_{2,3,4}}={{R}_{2}}+{{R}_{3}}+{{R}_{4}}=3+3+3=9\Omega $ --(4)
Now, ${{R}_{2,3,4}}$ and ${{R}_{5}}$ are in parallel.
Therefore using (2), we get, the equivalent resistance between $C$ and $D$ as
$\dfrac{1}{{{R}_{CD}}}=\dfrac{1}{{{R}_{5}}}+\dfrac{1}{{{R}_{2,3,4}}}=\dfrac{1}{3}+\dfrac{1}{9}=\dfrac{3+1}{9}=\dfrac{4}{9}$
$\therefore {{R}_{CD}}=\dfrac{9}{4}=2.25\Omega $ --(5)

(b) Equivalent resistance between $A$ and $B$
For the equivalent resistance between $A$ and $B$, we see that $\left( {{R}_{1}} \right),\left( \left( {{R}_{2}}+{{R}_{3}}+{{R}_{4}} \right)||{{R}_{5}} \right),{{R}_{6}}$ are in series, where
${{R}_{2}}+{{R}_{3}}+{{R}_{4}}={{R}_{2,3,4}}$ is the series combination of
${{R}_{2}},{{R}_{3}},{{R}_{4}}$.
Therefore using (1), we get, the equivalent resistance between $A$ and $B$ as
${{R}_{AB}}={{R}_{1}}+\left( {{R}_{2,3,4}}||{{R}_{5}} \right)+{{R}_{6}}$
$\therefore {{R}_{AB}}=3+2.25+3=8.25\Omega $ [Using (3) and (5)]
$\therefore {{R}_{AB}}=8.25\Omega $

Note: Students should take care between which two terminals the equivalent resistance is being asked to find because as is evident from the above question, the equivalent resistance changes depending upon between which two terminals the equivalent resistance is being calculated. Sometimes questions are given in such a way that the student jumps to conclusions and starts to calculate the equivalent resistance between one set of terminals but the question actually asks for the equivalent resistance between a completely different set of terminals.