
What is the formula for the radius of a semicircle?
Answer
443.1k+ views
Hint: We can write the formula for radius in terms of diameter, circumference or perimeter and area. We know that radius is half of diameter which gives the formula for radius in terms of diameter. The circumference of the semicircle is given by
Complete step by step solution:
We have to write the formula for radius of a semicircle. Let us see what a semicircle is. When a circle is divided into two equal halves along its diameter, we will get a semicircle. This is illustrated below.
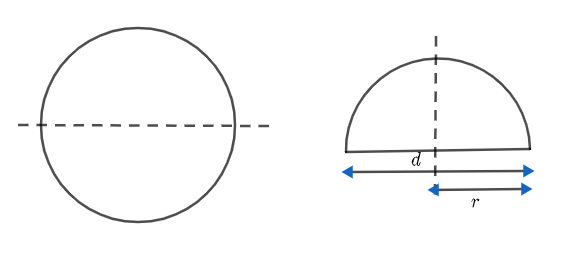
For a circle and semicircle, diameter and hence the radius remains the same.
The formula for radius of semicircle can be written in terms of diameter, circumference or perimeter and area.
Let us write the formula for radius in terms of diameter. We know that radius is half of diameter.
Where r is the radius and d is the diameter.
Now, we can write the radius in terms of circumference.
We can write the circumference of a semicircle as
Let us find the radius by taking
Now, let us write the formula for radius in terms of area of a semicircle. Area of a semicircle will be half of area of a circle. We know that area of a circle is
Let us take 2 from the RHS to the LHS.
The above equation can be written as
Let us take the square root.
Hence, the formulas for radius of semicircle are
Note: Students may think that the circumference of a semicircle will be half of that of a circle. This is not true. The area of the semicircle is half that of a circle. Students must note that the diameter and radius of a circle will be the same as that of a semicircle. This doesn’t mean that the formulas for radius of circle and semicircle will be the same.
Complete step by step solution:
We have to write the formula for radius of a semicircle. Let us see what a semicircle is. When a circle is divided into two equal halves along its diameter, we will get a semicircle. This is illustrated below.

For a circle and semicircle, diameter and hence the radius remains the same.
The formula for radius of semicircle can be written in terms of diameter, circumference or perimeter and area.
Let us write the formula for radius in terms of diameter. We know that radius is half of diameter.
Where r is the radius and d is the diameter.
Now, we can write the radius in terms of circumference.
We can write the circumference of a semicircle as
Let us find the radius by taking
Now, let us write the formula for radius in terms of area of a semicircle. Area of a semicircle will be half of area of a circle. We know that area of a circle is
Let us take 2 from the RHS to the LHS.
The above equation can be written as
Let us take the square root.
Hence, the formulas for radius of semicircle are
Note: Students may think that the circumference of a semicircle will be half of that of a circle. This is not true. The area of the semicircle is half that of a circle. Students must note that the diameter and radius of a circle will be the same as that of a semicircle. This doesn’t mean that the formulas for radius of circle and semicircle will be the same.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

The minimum age required for becoming the Prime Minister class 8 social science CBSE

A girl of height 90 cm is walking away from the base class 8 maths CBSE

How was Junagadh State merged into the Indian Unio class 8 social science CBSE

The length breadth and height of a room are 8 m 50 class 8 maths CBSE




