
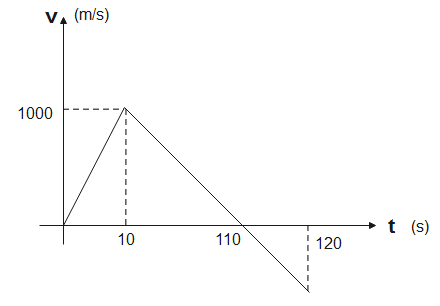
From the velocity-time graph of a rocket the maximum height attained by the rocket in

Answer
472.8k+ views
Hint: Rocket has maximum kinetic energy when it attains maximum velocity. By the law of conservation of mechanical energy, maximum kinetic energy=maximum potential energy. Substituting corresponding values, we can calculate maximum height. Convert between units as required.
Formula Used:
Complete step-by-step solution:
Velocity is defined as the displacement covered in unit time. Its SI unit is
According to the graph, the rocket moves in one direction. After it attains maximum velocity in one direction its velocity decreases till it reaches zero and then starts increasing in the opposite direction.
The formula for velocity is given by-
It reaches maximum velocity at the moment it attains its maximum velocity.
So, it can reach the maximum height when all of its kinetic energy is converted to potential energy. At maximum height total energy of the body is equal to its potential energy
Substituting given values for rocket in eq (2), we get,
Therefore, the maximum height attained by the rocket above the earth’s surface is
Note:
As height increases, potential energy increases and kinetic energy decreases. The potential energy is highest at maximum height and kinetic energy is lowest. The mechanical energy is the sum of kinetic and potential energy and it remains constant throughout. As the rocket is moving against gravity, the gravitational pull of the earth does work on the rocket which is stored as its potential energy.
Formula Used:
Complete step-by-step solution:

Velocity is defined as the displacement covered in unit time. Its SI unit is
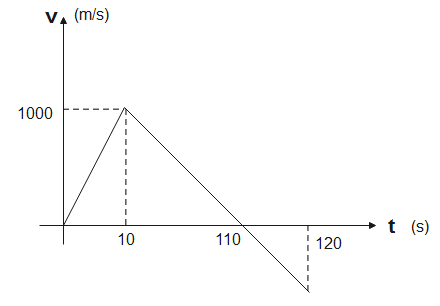
According to the graph, the rocket moves in one direction. After it attains maximum velocity in one direction its velocity decreases till it reaches zero and then starts increasing in the opposite direction.
The formula for velocity is given by-
It reaches maximum velocity at the moment it attains its maximum velocity.
So, it can reach the maximum height when all of its kinetic energy is converted to potential energy. At maximum height total energy of the body is equal to its potential energy
Substituting given values for rocket in eq (2), we get,
Therefore, the maximum height attained by the rocket above the earth’s surface is
Note:
As height increases, potential energy increases and kinetic energy decreases. The potential energy is highest at maximum height and kinetic energy is lowest. The mechanical energy is the sum of kinetic and potential energy and it remains constant throughout. As the rocket is moving against gravity, the gravitational pull of the earth does work on the rocket which is stored as its potential energy.
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




