
Given the electric field in the region E=2x


Answer
495.3k+ views
Hint: Electric flux is the rate of flow of electric field through a closed surface. In the given cubic surface which is closed we need to find the electric flux from the electric field, which can be calculated by a simple formula. The dot product of the electric field with the area enclosed gives us the electric flux.
Formula used:
The electric flux,
Complete answer:
Given,
Length of side of the cube = a
Electric Field = E=2x
The electric field given to us has only x component, so the flux will be present only in the x direction. To calculate the flux, we need to find the area of the region through which flux is flowing.
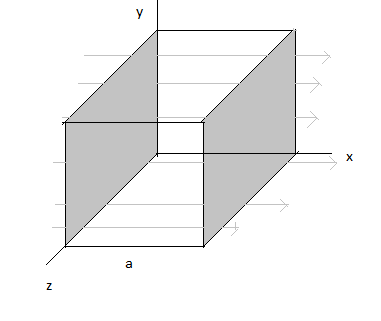
Fig: diagram showing electric field lines passing through the shaded regions in the x direction
The area of one side of the cube with side a =
The electric flux is given by the dot product of electric field and area of the enclosed surface of the cube.
The electric flux through the 2 surfaces of the cube with side a,
=2x
=2(a)
=2
Thus the electric flux in the region is given by 2
Additional Information:
Although electric fields cannot flow on their own, flux is the way of describing the electric field strength at any distance from the charge which causes the field. The Gauss law of Maxwell’s equation gives the relation between electric flux and charge. As electric flux exists in electrostatics, in electromagnetism, there is the existence of magnetic flux.
Note:
In this problem, we must keep in mind that the flux through the other 4 faces of the cube are 0 since the incoming flux is equal to outgoing flux across those faces in the absence of an electric field and hence the flux cancels to be 0. The area must necessarily be calculated of the region of which flux is calculated.
Formula used:
The electric flux,
Complete answer:
Given,
Length of side of the cube = a
Electric Field = E=2x
The electric field given to us has only x component, so the flux will be present only in the x direction. To calculate the flux, we need to find the area of the region through which flux is flowing.

Fig: diagram showing electric field lines passing through the shaded regions in the x direction
The area of one side of the cube with side a =
The electric flux is given by the dot product of electric field and area of the enclosed surface of the cube.
The electric flux through the 2 surfaces of the cube with side a,
=2x
=2(a)
=2
Thus the electric flux in the region is given by 2
Additional Information:
Although electric fields cannot flow on their own, flux is the way of describing the electric field strength at any distance from the charge which causes the field. The Gauss law of Maxwell’s equation gives the relation between electric flux and charge. As electric flux exists in electrostatics, in electromagnetism, there is the existence of magnetic flux.
Note:
In this problem, we must keep in mind that the flux through the other 4 faces of the cube are 0 since the incoming flux is equal to outgoing flux across those faces in the absence of an electric field and hence the flux cancels to be 0. The area must necessarily be calculated of the region of which flux is calculated.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




