
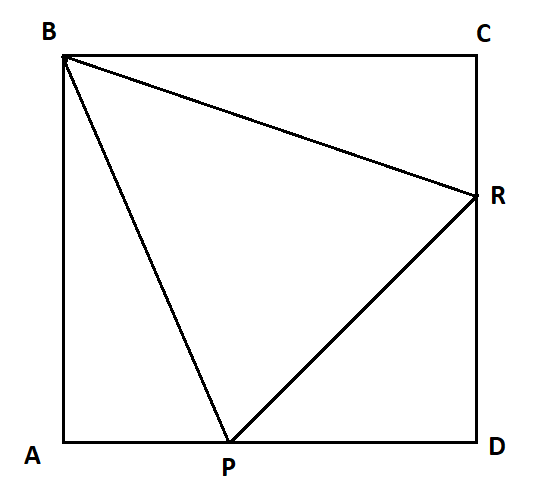
Given the equilateral triangle inscribed in a square of side s find the ratio of

Answer
397.2k+ views
Hint: The main objective is to find the ratio of two triangles.
Formula used:
Area of the triangle.
b is the base of the triangle and h is the height of the triangle.
Complete step by step answer:
Given,
To find
The
The angle
The value of BC is s.
Since
The perpendicular angle is
Subtract the values in the numerator
Divide the numerator and denominator
To find the side of CR of
Substitute the value of
We know
We know the formula for
Substitute the value of
Substitute
Substitute
Area of
Multiply the s terms
Now we need to calculate area of
Since we know that the side DR is equal to the square distance subtracts the Side CR
Substitute
Taking common out s
Evaluate the values in right side
Subtract the terms
Substitute DR in area of
Square the terms
We need to calculate
Cancel the same terms in the numerator and denominator
The value in the denominator goes to numerator by multiplication
We know
Squaring the terms, root and square get cancelled
Subtract the values in numerator
Divide the value in numerator and denominator
The ratio of
Note:
The side value should be taken correctly. Major mistakes can be made while choosing an angle as sometimes students forgets to take half of the angle. We should know that we can only proceed further because of the angle property of the equilateral triangle.
Formula used:
Area of the triangle.
b is the base of the triangle and h is the height of the triangle.
Complete step by step answer:
Given,
To find
The
The angle
The value of BC is s.
Since
The perpendicular angle is
Subtract the values in the numerator
Divide the numerator and denominator
To find the side of CR of
Substitute the value of
We know
We know the formula for
Substitute the value of
Substitute
Substitute
Area of
Multiply the s terms
Now we need to calculate area of
Since we know that the side DR is equal to the square distance subtracts the Side CR
Substitute
Taking common out s
Evaluate the values in right side
Subtract the terms
Substitute DR in area of
Square the terms
We need to calculate
Cancel the same terms in the numerator and denominator
The value in the denominator goes to numerator by multiplication
We know
Squaring the terms, root and square get cancelled
Subtract the values in numerator
Divide the value in numerator and denominator
The ratio of
Note:
The side value should be taken correctly. Major mistakes can be made while choosing an angle as sometimes students forgets to take half of the angle. We should know that we can only proceed further because of the angle property of the equilateral triangle.
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
Where did Netaji set up the INA headquarters A Yangon class 10 social studies CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

The British separated Burma Myanmar from India in 1935 class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

What are the public facilities provided by the government? Also explain each facility




