
How do you graph a linear inequality in only one variable?
Answer
454.2k+ views
Hint: We first take an arbitrary inequation as
Complete step-by-step solution:
We try to take an arbitrary linear inequality in only one variable as
The inequation
We first take some points for the x coordinates where
The values will be
We can take the y coordinate anything we want. The inequation is only based on the values of the x coordinates.
So, for y coordinate we take
The points are
We put these points in the graph to get
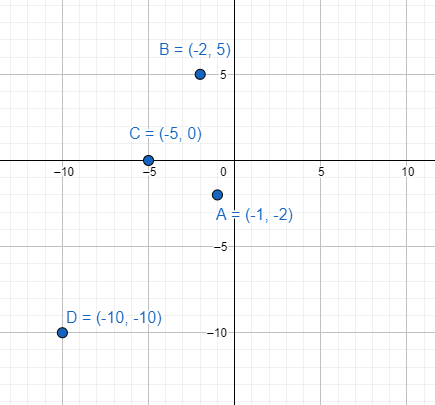
Based on the points we can measure the region.
Now we try to draw the line
Then we take all the x coordinates which have less value than
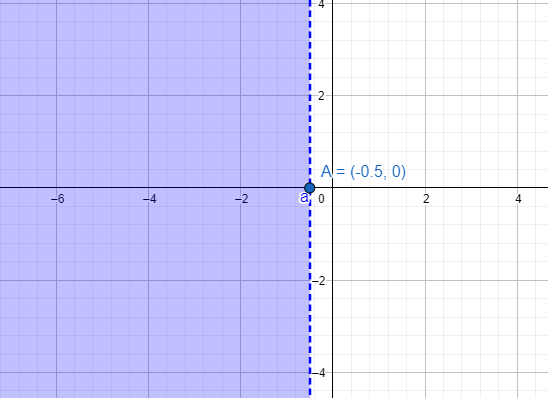
The dotted line is the equation
Note: We can also express the inequality as the interval system where
Complete step-by-step solution:
We try to take an arbitrary linear inequality in only one variable as
The inequation
We first take some points for the x coordinates where
The values will be
We can take the y coordinate anything we want. The inequation is only based on the values of the x coordinates.
So, for y coordinate we take
The points are
We put these points in the graph to get

Based on the points we can measure the region.
Now we try to draw the line
Then we take all the x coordinates which have less value than

The dotted line is the equation
Note: We can also express the inequality as the interval system where
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE





