
How do you graph
Answer
465.9k+ views
Hint: The given question describes the operation of addition/ subtraction/ multiplication/ division. Also, this problem involves substituting the
Complete step by step solution:
The given equation is shown below,
We would draw the graph for the above equation. As a first step, we would assume the
Let’s substitute
Let’s substitute
Let’s substitute
Let’s substitute
Let’s substitute
Let’s make a tabular column by using the
By using this table we can easily draw the following graph,
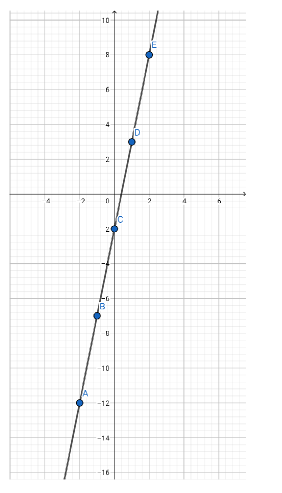
The above figure represents the equation
Note: This type of question involves the operation of addition/ subtraction/ multiplication/ division. In this type of question, we would assume the
1) When a negative number is multiplied with the negative number the answer becomes a
positive number
2) When a positive number is multiplied with the positive number the answer becomes a
positive number.
3) When a positive number is multiplied with the negative number the answer becomes a
negative number.
Complete step by step solution:
The given equation is shown below,
We would draw the graph for the above equation. As a first step, we would assume the
Let’s substitute
Let’s substitute
Let’s substitute
Let’s substitute
Let’s substitute
Let’s make a tabular column by using the
By using this table we can easily draw the following graph,

The above figure represents the equation
Note: This type of question involves the operation of addition/ subtraction/ multiplication/ division. In this type of question, we would assume the
1) When a negative number is multiplied with the negative number the answer becomes a
positive number
2) When a positive number is multiplied with the positive number the answer becomes a
positive number.
3) When a positive number is multiplied with the negative number the answer becomes a
negative number.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




