Question

If A, B and C are three points on a line, and B lies between A and C, then state whether the statement AB+BC=AC is true or not.

Answer 1

Hint: In this question, we are given that A, B and C are three points on a line, and B lies between A and C. As, we know that two segments will be equal if the coordinates of their end points are equal, we should try to find out the coordinates of the endpoints of AB+BC and AC and show that the coordinates are equal.
Complete step by step solution:
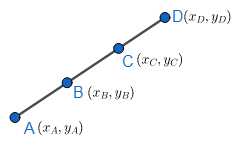
Let the following figure represent the situation geometrically. Now, let the coordinates of A be $\left( {{x}_{A}},{{y}_{A}} \right)$, that of B be \[\left( {{x}_{B}},{{y}_{B}} \right)\] , C be \[\left( {{x}_{C}},{{y}_{C}} \right)\] and that of D be \[\left( {{x}_{D}},{{y}_{D}} \right)\] …………………………(1.1)

Also, we know that when we add two line segments having one common endpoint, the coordinates of the resulting lines should be the coordinates of the points other than the common point, eg. Coordinates of endpoints upon addition of two segments PQ and QR where Q is the common point should be given by the coordinates of P and Q………………………………………..(1.2)
Therefore, (1.1) and (1.2), as B is the common endpoint, the starting point of AB+BC should be at A and the coordinates of other endpoint of AB+BC should the coordinates of C……………………(1.3)
Also, we know that two line segments are said to be equal if the coordinates of their end points are equal. Therefore, as A and C are the coordinates of end points of AC and from (1.3), as these coordinates match the coordinates of the endpoints of AB+BC, we can write AB+BC=AC which is the equation asked to be proved.

Note: We should note that we could use equation (1.2) in equation (1.3) only because B was a common end point between AB and BC. However, if there was no common endpoint then we could not have used the formula. However, the formula can still be used if AB and BC are given to be vectors, as vectors can be translated in parallel to match one end point of the two segments and thus we can then use (1.2) in that case.