
If
(a) 5
(b) 7
(c) 2
(d) 12
Answer
532.2k+ views
Hint: Use the formula for union of two sets that is
Here, we are given two sets A and B such that
Before proceeding with the question, we must know some of the terminologies related to sets.
First of all, a ‘set’ is a collection of well-defined and distinct objects. The most basic property of a set is that it has elements. The number of elements of a set, say A is shown by
Here, in the question we have
Now, a ‘subset’ is a set which is contained in another set. We can also put it as, if we have a set P which is a subset of another set Q, then P is contained in Q as all the elements of set P are elements of Q. This relationship is shown by
We can show it diagrammatically as,
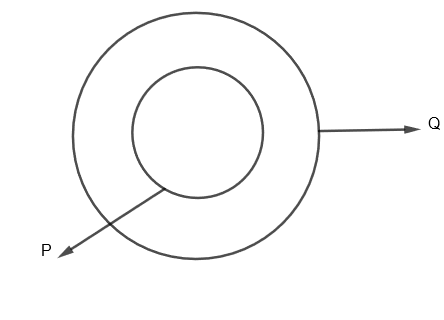
Here,
In question, we are given that
Now, union of two sets say P and Q is the set of elements which are in P, in Q or both P and Q. For example, if P = {1, 3, 5, 7} and Q = {1, 2, 4, 6, 7}, then union of P and Q which is shown as
Diagrammatically, the shaded portion is
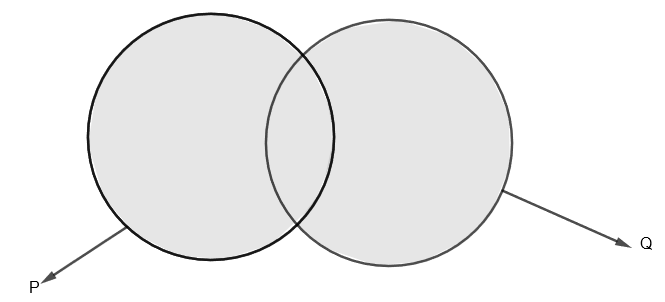
The formula for
Here,
Now, in the given question, we have to find
We can show
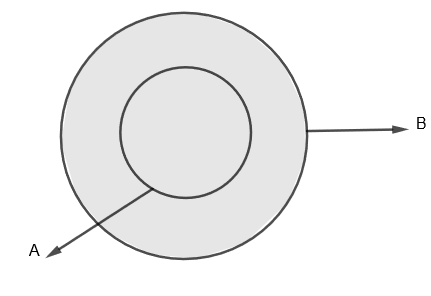
Here,
Here, we can see that the portion common to the set A and B that is
As we know that
Since, we have found that
Therefore we get,
By putting the values of n (A) and n (B), we get,
Therefore, we get
Hence, option (b) is correct.
Note: Students must note that whenever
Here, we are given two sets A and B such that
Before proceeding with the question, we must know some of the terminologies related to sets.
First of all, a ‘set’ is a collection of well-defined and distinct objects. The most basic property of a set is that it has elements. The number of elements of a set, say A is shown by
Here, in the question we have
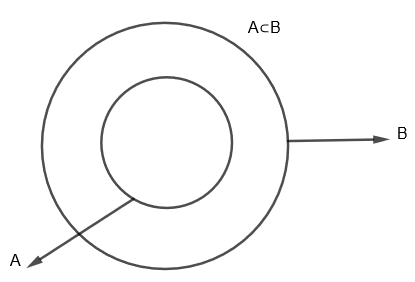
Now, a ‘subset’ is a set which is contained in another set. We can also put it as, if we have a set P which is a subset of another set Q, then P is contained in Q as all the elements of set P are elements of Q. This relationship is shown by
We can show it diagrammatically as,

Here,
In question, we are given that

Now, union of two sets say P and Q is the set of elements which are in P, in Q or both P and Q. For example, if P = {1, 3, 5, 7} and Q = {1, 2, 4, 6, 7}, then union of P and Q which is shown as
Diagrammatically, the shaded portion is

The formula for
Here,
Now, in the given question, we have to find
We can show

Here,
Here, we can see that the portion common to the set A and B that is
As we know that
Since, we have found that
Therefore we get,
By putting the values of n (A) and n (B), we get,
Therefore, we get
Hence, option (b) is correct.
Note: Students must note that whenever
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE




