
If \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\], then \[f\left( x \right) = \]
(a) \[x - 1\]
(b) \[x + 1\]
(c) \[x\]
(d) \[x + 2\]
Answer
456.9k+ views
Hint: Here, we need to find the value of \[f\left( x \right)\]. We will simplify the left hand side using a long division method. Then, we will use the division algorithm and rewrite the expression. Finally, we will compare the equation obtained with the given equation to find the value of \[f\left( x \right)\].
Formula Used:
The division algorithm states that if \[p\left( x \right)\] and \[g\left( x \right)\] are two polynomials where \[g\left( x \right) \ne 0\], then there are two polynomials \[q\left( x \right)\] and \[r\left( x \right)\] such that \[p\left( x \right) = q\left( x \right) \times g\left( x \right) + r\left( x \right)\]. Here, \[p\left( x \right)\] is the dividend, \[g\left( x \right)\] is the divisor, \[q\left( x \right)\] is the quotient, and \[r\left( x \right)\] is the remainder.
Complete step-by-step answer:
First, we will use a long division method to divide \[{x^3} - 6{x^2} + 10x - 2\] by \[{x^2} - 5x + 6\].
Therefore, we get
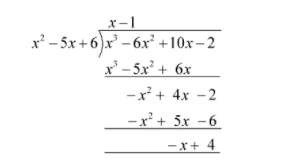
We can observe that when \[{x^3} - 6{x^2} + 10x - 2\] is divided by \[{x^2} - 5x + 6\] using long division method, the quotient is \[x - 1\], and the remainder is \[ - x + 4\].
Now, we will use the division algorithm.
The division algorithm states that if \[p\left( x \right)\] and \[g\left( x \right)\] are two polynomials where \[g\left( x \right) \ne 0\], then there are two polynomials \[q\left( x \right)\] and \[r\left( x \right)\] such that \[p\left( x \right) = q\left( x \right) \times g\left( x \right) + r\left( x \right)\]. Here, \[p\left( x \right)\] is the dividend, \[g\left( x \right)\] is the divisor, \[q\left( x \right)\] is the quotient, and \[r\left( x \right)\] is the remainder.
Thus, we get
\[p\left( x \right) = {x^3} - 6{x^2} + 10x - 2\]
\[q\left( x \right) = x - 1\]
\[r\left( x \right) = - x + 4\]
\[g\left( x \right) = {x^2} - 5x + 6\]
Substituting \[p\left( x \right) = {x^3} - 6{x^2} + 10x - 2\], \[q\left( x \right) = x - 1\], \[r\left( x \right) = - x + 4\], and \[g\left( x \right) = {x^2} - 5x + 6\] in the division algorithm, we get
\[ \Rightarrow {x^3} - 6{x^2} + 10x - 2 = \left( {x - 1} \right)\left( {{x^2} - 5x + 6} \right) + \left( { - x + 4} \right)\]
Dividing both sides by \[{x^2} - 5x + 6\], we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 + \left( {\dfrac{{ - x + 4}}{{{x^2} - 5x + 6}}} \right)\]
Rewriting the expression, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left( {\dfrac{{x - 4}}{{{x^2} - 5x + 6}}} \right)\]
Factoring the denominator, we get
\[\begin{array}{l} \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left( {\dfrac{{x - 4}}{{{x^2} - 3x - 2x + 6}}} \right)\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left[ {\dfrac{{x - 4}}{{x\left( {x - 3} \right) - 2\left( {x - 3} \right)}}} \right]\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left[ {\dfrac{{x - 4}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\end{array}\]
Multiplying and dividing the fraction by 2, we get
\[\begin{array}{l} \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{2}{2}\left[ {\dfrac{{x - 4}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{2\left( {x - 4} \right)}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\end{array}\]
Multiplying the above terms using the distributive property, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{2x - 8}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\]
Rewriting the numerator, we get
\[\begin{array}{l} \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{x + x - 3 - 3 - 2}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{x - 3 + x - 2 - 3}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\end{array}\]
Separating the fractions with the same denominator, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{x - 3}}{{\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{x - 2}}{{\left( {x - 3} \right)\left( {x - 2} \right)}} - \dfrac{3}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\]
Simplifying the expressions, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{1}{{x - 2}} + \dfrac{1}{{x - 3}} - \dfrac{3}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\]
Multiplying the above terms using the distributive property, we get\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{{2\left( {x - 2} \right)}} - \dfrac{1}{{2\left( {x - 3} \right)}} + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}}\]
Rearranging the terms of the expression, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 3} \right)}}\]
It is given that \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\].
Here, \[A\] and \[B\] are constants.
Therefore, from the equations \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 3} \right)}}\] and \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\], we get
\[ \Rightarrow x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 3} \right)}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\]
Comparing the terms of the expressions, we get
\[x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} = f\left( x \right)\]
\[\Rightarrow - \dfrac{1}{2} = A\]
\[\Rightarrow - \dfrac{1}{2} = B\]
Therefore, we get the value of \[f\left( x \right)\] as \[x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}}\].
Thus, none of the given options are correct.
Note: We have used the distributive law of multiplication to multiply some expressions in the solution. The distributive law of multiplication states that \[a\left( {b + c} \right) = a \cdot b + a \cdot c\]. Here for finding the value of \[f\left( x \right)\] it is important for us to find the value of A and B.
Formula Used:
The division algorithm states that if \[p\left( x \right)\] and \[g\left( x \right)\] are two polynomials where \[g\left( x \right) \ne 0\], then there are two polynomials \[q\left( x \right)\] and \[r\left( x \right)\] such that \[p\left( x \right) = q\left( x \right) \times g\left( x \right) + r\left( x \right)\]. Here, \[p\left( x \right)\] is the dividend, \[g\left( x \right)\] is the divisor, \[q\left( x \right)\] is the quotient, and \[r\left( x \right)\] is the remainder.
Complete step-by-step answer:
First, we will use a long division method to divide \[{x^3} - 6{x^2} + 10x - 2\] by \[{x^2} - 5x + 6\].
Therefore, we get
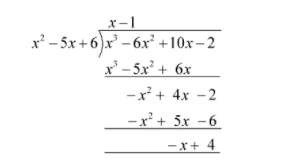
We can observe that when \[{x^3} - 6{x^2} + 10x - 2\] is divided by \[{x^2} - 5x + 6\] using long division method, the quotient is \[x - 1\], and the remainder is \[ - x + 4\].
Now, we will use the division algorithm.
The division algorithm states that if \[p\left( x \right)\] and \[g\left( x \right)\] are two polynomials where \[g\left( x \right) \ne 0\], then there are two polynomials \[q\left( x \right)\] and \[r\left( x \right)\] such that \[p\left( x \right) = q\left( x \right) \times g\left( x \right) + r\left( x \right)\]. Here, \[p\left( x \right)\] is the dividend, \[g\left( x \right)\] is the divisor, \[q\left( x \right)\] is the quotient, and \[r\left( x \right)\] is the remainder.
Thus, we get
\[p\left( x \right) = {x^3} - 6{x^2} + 10x - 2\]
\[q\left( x \right) = x - 1\]
\[r\left( x \right) = - x + 4\]
\[g\left( x \right) = {x^2} - 5x + 6\]
Substituting \[p\left( x \right) = {x^3} - 6{x^2} + 10x - 2\], \[q\left( x \right) = x - 1\], \[r\left( x \right) = - x + 4\], and \[g\left( x \right) = {x^2} - 5x + 6\] in the division algorithm, we get
\[ \Rightarrow {x^3} - 6{x^2} + 10x - 2 = \left( {x - 1} \right)\left( {{x^2} - 5x + 6} \right) + \left( { - x + 4} \right)\]
Dividing both sides by \[{x^2} - 5x + 6\], we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 + \left( {\dfrac{{ - x + 4}}{{{x^2} - 5x + 6}}} \right)\]
Rewriting the expression, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left( {\dfrac{{x - 4}}{{{x^2} - 5x + 6}}} \right)\]
Factoring the denominator, we get
\[\begin{array}{l} \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left( {\dfrac{{x - 4}}{{{x^2} - 3x - 2x + 6}}} \right)\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left[ {\dfrac{{x - 4}}{{x\left( {x - 3} \right) - 2\left( {x - 3} \right)}}} \right]\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \left[ {\dfrac{{x - 4}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\end{array}\]
Multiplying and dividing the fraction by 2, we get
\[\begin{array}{l} \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{2}{2}\left[ {\dfrac{{x - 4}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{2\left( {x - 4} \right)}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\end{array}\]
Multiplying the above terms using the distributive property, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{2x - 8}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\]
Rewriting the numerator, we get
\[\begin{array}{l} \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{x + x - 3 - 3 - 2}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\\ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{x - 3 + x - 2 - 3}}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\end{array}\]
Separating the fractions with the same denominator, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{{x - 3}}{{\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{x - 2}}{{\left( {x - 3} \right)\left( {x - 2} \right)}} - \dfrac{3}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\]
Simplifying the expressions, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{2}\left[ {\dfrac{1}{{x - 2}} + \dfrac{1}{{x - 3}} - \dfrac{3}{{\left( {x - 3} \right)\left( {x - 2} \right)}}} \right]\]
Multiplying the above terms using the distributive property, we get\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 - \dfrac{1}{{2\left( {x - 2} \right)}} - \dfrac{1}{{2\left( {x - 3} \right)}} + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}}\]
Rearranging the terms of the expression, we get
\[ \Rightarrow \dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 3} \right)}}\]
It is given that \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\].
Here, \[A\] and \[B\] are constants.
Therefore, from the equations \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 3} \right)}}\] and \[\dfrac{{{x^3} - 6{x^2} + 10x - 2}}{{{x^2} - 5x + 6}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\], we get
\[ \Rightarrow x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 2} \right)}} + \dfrac{{ - \dfrac{1}{2}}}{{\left( {x - 3} \right)}} = f\left( x \right) + \dfrac{A}{{x - 2}} + \dfrac{B}{{x - 3}}\]
Comparing the terms of the expressions, we get
\[x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}} = f\left( x \right)\]
\[\Rightarrow - \dfrac{1}{2} = A\]
\[\Rightarrow - \dfrac{1}{2} = B\]
Therefore, we get the value of \[f\left( x \right)\] as \[x - 1 + \dfrac{3}{{2\left( {x - 3} \right)\left( {x - 2} \right)}}\].
Thus, none of the given options are correct.
Note: We have used the distributive law of multiplication to multiply some expressions in the solution. The distributive law of multiplication states that \[a\left( {b + c} \right) = a \cdot b + a \cdot c\]. Here for finding the value of \[f\left( x \right)\] it is important for us to find the value of A and B.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
Assertion The planet Neptune appears blue in colour class 10 social science CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Change the following sentences into negative and interrogative class 10 english CBSE

Write a letter to the principal requesting him to grant class 10 english CBSE

The capital of British India was transferred from Calcutta class 10 social science CBSE




