
If
Answer
484.2k+ views
Hint: In this question, we have to find out the required trigonometric expression’s value from the given equation.
We need to first use the trigonometric formulas to bring the given equation in a shorter form so that we can find out the value of θ from the given equation then putting the value of θ in the given expression we will get the solution.
Trigonometric formula:
Complete step-by-step solution:
The given trigonometric equation is
We need to find out the value of
Now, we have to first find out the value of θ from the given equation.
We have,
We know,
Putting the formula in the given equation we get,
By cross multiplication we get,
Solving the equation we get,
Squaring we get,
We know,
Hence,
Hence, the value of
Note: Sin Cos formulas are based on sides of the right-angled triangle. Sin and Cos are basic trigonometric functions along with tan function, in trigonometry. Sine of angle is equal to the ratio of opposite side and hypotenuse whereas cosine of an angle is equal to ratio of adjacent side and hypotenuse.
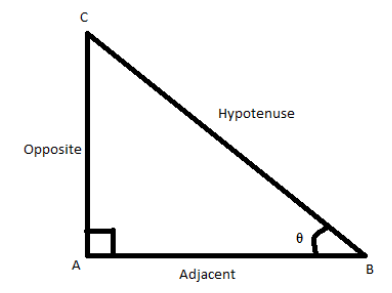
In mathematics, the trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others. The most widely used trigonometric functions are the sine, the cosine, and the tangent.
We need to first use the trigonometric formulas to bring the given equation in a shorter form so that we can find out the value of θ from the given equation then putting the value of θ in the given expression we will get the solution.
Trigonometric formula:
Complete step-by-step solution:
The given trigonometric equation is
We need to find out the value of
Now, we have to first find out the value of θ from the given equation.
We have,
We know,
Putting the formula in the given equation we get,
By cross multiplication we get,
Solving the equation we get,
Squaring we get,
We know,
Hence,
Hence, the value of
Note: Sin Cos formulas are based on sides of the right-angled triangle. Sin and Cos are basic trigonometric functions along with tan function, in trigonometry. Sine of angle is equal to the ratio of opposite side and hypotenuse whereas cosine of an angle is equal to ratio of adjacent side and hypotenuse.

In mathematics, the trigonometric functions are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in all sciences that are related to geometry, such as navigation, solid mechanics, celestial mechanics, geodesy, and many others. The most widely used trigonometric functions are the sine, the cosine, and the tangent.
Recently Updated Pages
Master Class 4 Maths: Engaging Questions & Answers for Success

Master Class 4 English: Engaging Questions & Answers for Success

Master Class 4 Science: Engaging Questions & Answers for Success

Class 4 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Trending doubts
What is the difference between resemblance and sem class 12 social science CBSE

Draw ray diagrams each showing i myopic eye and ii class 12 physics CBSE

Why do the transition elements have higher enthalpies class 12 chemistry CBSE

What are the advantages of parallel combination over class 12 physics CBSE

What are the causes of overloading class 12 physics CBSE

There is hypertension due to smoking Give scientific class 12 biology CBSE




