Question

If \[\sqrt {\dfrac{{1 + \sin A}}{{1 - \sin A}}} = \sec A + \tan A\], then, the quadrant in which the angle A lies are.
A. I, II
B. II, III
C. I, IV
D. III, V

Answer 1

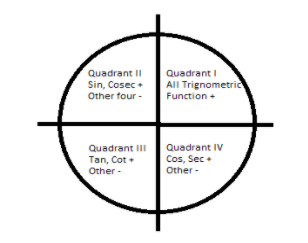
Hint: When two lines, one real axis and the other imaginary axis passes perpendicular through a circle, the circle is divided into four quadrants. Into quadrant I, where both x and y-axis are positive, Quadrant II, here x-axis is negative, and the y-axis is positive, Quadrant III here both the x-axis and y-axis are negative, and in Quadrant IV x-axis is positive, and the y-axis is negative.
In the case of the trigonometric functions in quadrant I, all the functions are positive, in Quadrant II, Sin and Cosec functions are positive, and other functions are negative, in Quadrant III, tan and cot functions are positive and other are negative, and in the case of Quadrant IV, Cos and Sec functions are positive and other being negative.

Complete step by step solution: \[\sqrt {\dfrac{{1 + \sin A}}{{1 - \sin A}}} = \sec A + \tan A\]
One of the methods to remove the square root is the rationalization where the numerator and the denominator are multiplied by the same rational number; hence we will rationalize the LHS of the given function:
\[
  \sqrt {\dfrac{{1 + \sin A}}{{1 - \sin A}}} = \sqrt {\dfrac{{\left( {1 + \sin A} \right)\left( {1 + \sin A} \right)}}{{\left( {1 - \sin A} \right)\left( {1 + \sin A} \right)}}} \\
   = \sqrt {\dfrac{{{{\left( {1 + \sin A} \right)}^2}}}{{\left( {1 - {{\sin }^2}A} \right)}}} \\
   = \dfrac{{\left( {1 + \sin A} \right)}}{{\sqrt {\left( {{{\cos }^2}A} \right)} }}{\text{ }}\left[ {\because {{\sin }^2}A + {{\cos }^2}A = 1} \right] \\
   = \dfrac{{\left( {1 + \sin A} \right)}}{{\left| {\cos A} \right|}} \\
 \]
Hence, we have got the LHS as: \[\dfrac{{\left( {1 + \sin A} \right)}}{{\left| {\cos A} \right|}}\]
We know\[\sqrt {\left( {{{\cos }^2}A} \right)} = \left| {\cos A} \right| = \pm \cos A\], hence we put the value \[ + \cos A\]and \[ - \cos A\]to check the function:
\[\dfrac{{\left( {1 + \sin A} \right)}}{{\left| {\cos A} \right|}} = \dfrac{1}{{ + \cos A}} + \dfrac{{\sin A}}{{ + \cos A}} = \sec A + \tan A - - - - (i)\]
\[\dfrac{{\left( {1 + \sin A} \right)}}{{\left| {\cos A} \right|}} = \dfrac{1}{{ - \cos A}} + \dfrac{{\sin A}}{{ - \cos A}} = - \sec A - \tan A - - - - (ii)\]
Hence we can see in equation (i) \[ + \cos A\] satisfy the given equation, \[\cos A\] is positive only in quadrant I and quadrant IV.
Option C is correct.

Note: The co-function identities show the relationship between the sin, cos, tan, cosine, sec, and cot function. The value of the trigonometric function for an angle is equal to the value of the cofunction of the complement.