
If the length of a common internal tangent to two circles is 7 and that of a common external tangent is 11, then the product of the radii of the two circles is:
A. 36
B. 9
C. 18
D. 4
Answer
473.1k+ views
1 likes
Hint: The length of a direct (external) common tangent to two circles is
The length of a transverse (internal) common tangent to two circles is
Form two equations. We cannot find the values of
Complete step by step answer:
The two tangents be
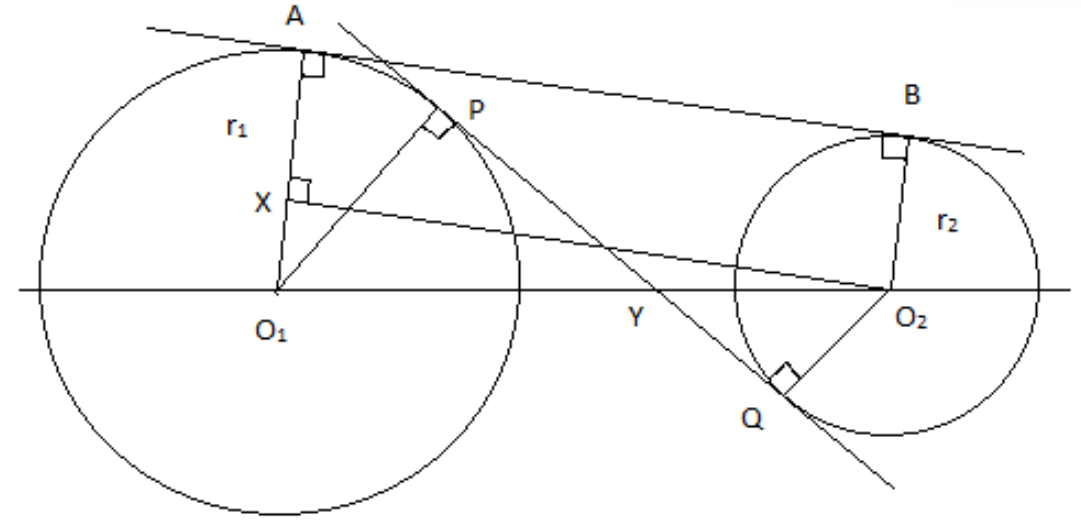
Using the formula for the length of the direct common tangent, we have the following equation:
⇒
⇒
Using the formula for the length of the transverse common tangent, we have the following equation:
⇒
⇒
Subtracting equation (2) from equation (1), we get:
⇒
Dividing both sides by 4, gives us:
⇒
Therefore, the answer is C. 18.
Note: Both the direct tangents are equal in length. Both the transverse tangents are also equal.
Direct tangents are longer than the transverse tangents.
The tangents to a circle are perpendicular to the radius of the circle at the point of contact.
A line
The length of a transverse (internal) common tangent to two circles is
Form two equations. We cannot find the values of
Complete step by step answer:
The two tangents be

Using the formula for the length of the direct common tangent, we have the following equation:
⇒
⇒
Using the formula for the length of the transverse common tangent, we have the following equation:
⇒
⇒
Subtracting equation (2) from equation (1), we get:
⇒
Dividing both sides by 4, gives us:
⇒
Therefore, the answer is C. 18.
Note: Both the direct tangents are equal in length. Both the transverse tangents are also equal.
Direct tangents are longer than the transverse tangents.
The tangents to a circle are perpendicular to the radius of the circle at the point of contact.
A line
Recently Updated Pages
Express the following as a fraction and simplify a class 7 maths CBSE

The length and width of a rectangle are in ratio of class 7 maths CBSE

The ratio of the income to the expenditure of a family class 7 maths CBSE

How do you write 025 million in scientific notatio class 7 maths CBSE

How do you convert 295 meters per second to kilometers class 7 maths CBSE

Write the following in Roman numerals 25819 class 7 maths CBSE

Trending doubts
How many ounces are in 500 mL class 8 maths CBSE

Summary of the poem Where the Mind is Without Fear class 8 english CBSE

Advantages and disadvantages of science

10 slogans on organ donation class 8 english CBSE

The LCM and HCF of two rational numbers are equal Then class 8 maths CBSE

In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE




