
If the power factor in a circuit is unity, then the impedance of the circuit is
A. Inductive
B. Capacitive
C. Partially inductive and partially capacitive
D. Resistive
Answer
496.5k+ views
Hint: It is important to know the definition of power factor for solving this question. The power factor is defined as the ratio of the real power absorbed by the load to the apparent power flowing in the circuit. It is a dimensionless number ranging from -1 to +1.
Complete step by step answer:
Consider the following LRC circuit connected to an AC source of voltage
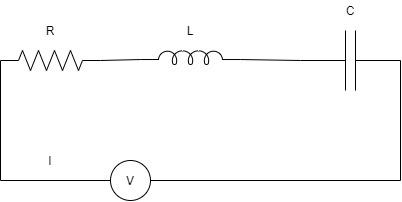
The impedance, Z is defined as the resistance offered to the flow of alternating current I through the entire circuit. Here, in the figure, the impedance Z is the vector sum of the reactances of the individual resistance, inductor, and the capacitor.
Capacitive reactance,
Inductive reactance,
Resistance is
Impedance,
These quantities cannot be added in a scalar manner since they have different phases. We have to add these values through vector addition by drawing the impedance triangle with
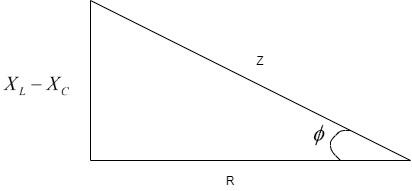
Thus, we can see that the Z is the vector sum of the reactance and the resistance as the triangle.
Here, the value of
Power factor = Real power / Apparent power
Power factor =
Therefore, from the figure, we can write that, Power factor =
For the power factor to be one,
where,
Substituting in the impedance equation, we get –
Here, we see that inductive and capacitive reactance is equal and thus, they cancel out each other. When they cancel out, only the resistance stands out.
Thus, the circuit where only resistance acts is called a resistive circuit. Hence, the correct option is Option D.
Note:
Some students may get confused as to why we have taken
Complete step by step answer:
Consider the following LRC circuit connected to an AC source of voltage

The impedance, Z is defined as the resistance offered to the flow of alternating current I through the entire circuit. Here, in the figure, the impedance Z is the vector sum of the reactances of the individual resistance, inductor, and the capacitor.
Capacitive reactance,
Inductive reactance,
Resistance is
Impedance,
These quantities cannot be added in a scalar manner since they have different phases. We have to add these values through vector addition by drawing the impedance triangle with

Thus, we can see that the Z is the vector sum of the reactance and the resistance as the triangle.
Here, the value of
Power factor = Real power / Apparent power
Power factor =
Therefore, from the figure, we can write that, Power factor =
For the power factor to be one,
where,
Substituting in the impedance equation, we get –
Here, we see that inductive and capacitive reactance is equal and thus, they cancel out each other. When they cancel out, only the resistance stands out.
Thus, the circuit where only resistance acts is called a resistive circuit. Hence, the correct option is Option D.
Note:
Some students may get confused as to why we have taken
Recently Updated Pages
Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Class 10 Question and Answer - Your Ultimate Solutions Guide

Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

What is the difference between resemblance and sem class 12 social science CBSE




