Question

If the triangle ABC is similar to the triangle PQR. Then find the value of PQ.

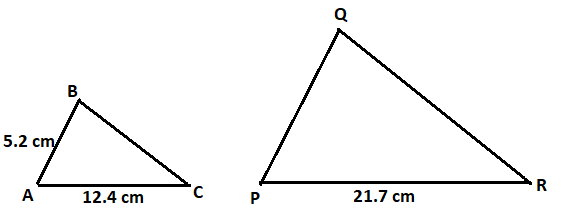
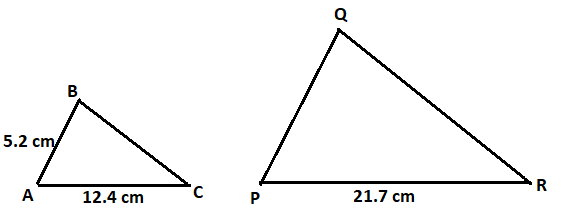
Answer 1

Hint: We can use BPT (basic proportionality theorem) which states that, if a line is parallel to a side of a triangle which intersects the other sides into two distinct points, then the line divides those sides in proportion ratio. Like, if triangles LMN and XYZ are similar triangles, we can say that \[\angle L = \angle X,{\text{ }}\angle M = \angle Y,{\text{ }}\angle N = \angle Z\] and \[\dfrac{{LM}}{{XY}} = \dfrac{{MN}}{{YZ}} = \dfrac{{NL}}{{ZX}}\] because triangles LMN and XYZ are similar.

Complete step-by-step solution -
As we know that the triangle ABC and triangle PQR are similar to each other.
Now as we know that if two triangles are similar then their corresponding sides and angles are also similar.
Like if two triangles LMN and XYZ are similar then the corresponding angles are equal i.e. \[\angle L = \angle X\], \[\angle M = \angle Y\] and \[\angle N = \angle Z\].
And corresponding sides must be proportional to each other or we can say that the ratio of each corresponding side is equal.
So, \[\dfrac{{LM}}{{XY}} = \dfrac{{MN}}{{YZ}} = \dfrac{{NL}}{{ZX}}\]
So, now in triangle ABC and PQR.
\[\angle A = \angle P\], \[\angle B = \angle Q\] and \[\angle C = \angle R\].
And, \[\dfrac{{AB}}{{PQ}} = \dfrac{{BC}}{{QR}} = \dfrac{{CA}}{{RP}}\]
As we can see from the above figure that AB = 5.2 cm , CA = 12.4 cm and RP = 21.7 cm.
So, \[\dfrac{{AB}}{{PQ}} = \dfrac{{5.2}}{{PQ}}\] and \[\dfrac{{CA}}{{RP}} = \dfrac{{12.4}}{{21.7}}\]
And as we know that,
\[\dfrac{{AB}}{{PQ}} = \dfrac{{CA}}{{RP}}\]
So, \[\dfrac{{5.2}}{{PQ}} = \dfrac{{12.4}}{{21.7}}\] (1)
Now to find the value of PQ. Cross multiplying both sides of the equation 1. We get,
\[PQ = 5.2 \times \dfrac{{21.7}}{{12.4}} = \dfrac{{112.84}}{{12.4}} = 9.1cm\]
So, the length of side PQ of the triangle PQR is 9.1 cm.

Note: Whenever we come up with this type of problem then we should remember that it is if two triangles are similar then their corresponding sides and angles may or may not be of equal length. So, here using the BPT theorem we have equated the ratio of sides AB and PQ with the ratio of sides CA and RP. And then put the value of AB, CA and Rp in the equation. After cross multiplying the equation we will get the required length of PQ. This will be the easiest and efficient way to find the solution of the problem.