Question

If we are given two vectors as \[\overset{\to }{\mathop{a}}\,=2\overset{\wedge }{\mathop{i}}\,-3\overset{\wedge }{\mathop{j}}\,+4\overset{\wedge }{\mathop{k}}\,\] and \[\overset{\to }{\mathop{b}}\,=\overset{\wedge }{\mathop{i}}\,+\overset{\wedge }{\mathop{j}}\,+\overset{\wedge }{\mathop{k}}\,\], then find \[\overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,\].

Answer 1

Hint: To find \[\overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,\], that is the dot product of vectors \[\overset{\to }{\mathop{a}}\,\] and \[\overset{\to }{\mathop{b}}\,\], multiply the coefficients of \[\overset{\wedge }{\mathop{i}}\,,\overset{\wedge }{\mathop{j}}\,\] and \[\overset{\wedge }{\mathop{k}}\,\] of vector a with the corresponding coefficients of \[\overset{\wedge }{\mathop{i}}\,,\overset{\wedge }{\mathop{j}}\,\] and \[\overset{\wedge }{\mathop{k}}\,\] of vector b. Use the fact that the dot product of two perpendicular vectors is 0, so \[\overset{\wedge }{\mathop{i}}\,.\overset{\wedge }{\mathop{j}}\,=\overset{\wedge }{\mathop{j}}\,.\overset{\wedge }{\mathop{i}}\,=0,\overset{\wedge }{\mathop{j}}\,.\overset{\wedge }{\mathop{k}}\,=\overset{\wedge }{\mathop{k}}\,.\overset{\wedge }{\mathop{j}}\,=0\] and \[\overset{\wedge }{\mathop{i}}\,.\overset{\wedge }{\mathop{k}}\,=\overset{\wedge }{\mathop{k}}\,.\overset{\wedge }{\mathop{i}}\,=0\].

Complete step by step solution:
Here, we have been provided with two vectors, \[\overset{\to }{\mathop{a}}\,=2\overset{\wedge }{\mathop{i}}\,-3\overset{\wedge }{\mathop{j}}\,+4\overset{\wedge }{\mathop{k}}\,\] and \[\overset{\to }{\mathop{b}}\,=\overset{\wedge }{\mathop{i}}\,+\overset{\wedge }{\mathop{j}}\,+\overset{\wedge }{\mathop{k}}\,\], and we are asked to find the value of the expression \[\overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,\], i.e., the dot product of the two vectors.
Now, the dot product of two vectors is defined as the product of the magnitude of two vectors and the cosine of the angle between them. For example: - let us consider two vectors, \[\overset{\to }{\mathop{x}}\,\] and \[\overset{\to }{\mathop{y}}\,\] and the angle between them is \[\theta \]. So, the dot product of these vectors is given as: -

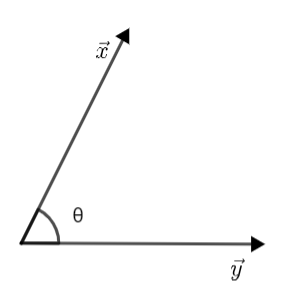
\[\Rightarrow \overrightarrow{x}.\overrightarrow{y}=xy\cos \theta \]
Here, x denotes the magnitude of \[\overrightarrow{x}\] and similarly y denotes the magnitude of \[\overrightarrow{y}\]. Now, one thing you may note is that when the two vectors are perpendicular, i.e., \[\theta ={{90}^{\circ }}\], then the dot product of two vectors will become zero. This is because \[\cos {{90}^{\circ }}=0\].
Now, we know that \[\overset{\wedge }{\mathop{i}}\,,\overset{\wedge }{\mathop{j}}\,\] and \[\overset{\wedge }{\mathop{k}}\,\] are perpendicular to each as they represent unit vectors along x, y and z axis respectively. So, we have,
\[\Rightarrow \]\[\overset{\wedge }{\mathop{i}}\,.\overset{\wedge }{\mathop{j}}\,=\overset{\wedge }{\mathop{j}}\,.\overset{\wedge }{\mathop{i}}\,=0,\overset{\wedge }{\mathop{j}}\,.\overset{\wedge }{\mathop{k}}\,=\overset{\wedge }{\mathop{k}}\,.\overset{\wedge }{\mathop{j}}\,=0\] and \[\overset{\wedge }{\mathop{i}}\,.\overset{\wedge }{\mathop{k}}\,=\overset{\wedge }{\mathop{k}}\,.\overset{\wedge }{\mathop{i}}\,=0\]
That means we have to take the product of \[\overset{\wedge }{\mathop{i}}\,,\overset{\wedge }{\mathop{j}}\,\] and \[\overset{\wedge }{\mathop{k}}\,\] of one vector with the corresponding \[\overset{\wedge }{\mathop{i}}\,,\overset{\wedge }{\mathop{j}}\,\] and \[\overset{\wedge }{\mathop{k}}\,\] of the second vector. Here, \[\overset{\wedge }{\mathop{i}}\,.\overset{\wedge }{\mathop{i}}\,=\overset{\wedge }{\mathop{j}}\,.\overset{\wedge }{\mathop{j}}\,=\overset{\wedge }{\mathop{k}}\,.\overset{\wedge }{\mathop{k}}\,=1\] because in these three cases \[\theta ={{0}^{\circ }}\] and we know that \[\cos {{0}^{\circ }}=1\].
Let us come to the question, considering the dot product of vectors a and b, we get,
\[\begin{align}
  & \Rightarrow \overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,=\left( 2\overset{\wedge }{\mathop{i}}\,-3\overset{\wedge }{\mathop{j}}\,+4\overset{\wedge }{\mathop{k}}\, \right).\left( \overset{\wedge }{\mathop{i}}\,+\overset{\wedge }{\mathop{j}}\,+\overset{\wedge }{\mathop{k}}\, \right) \\
 & \Rightarrow \overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,=\left( 2\times 1 \right)+\left( -3 \right)\times 1+4\times 1 \\
 & \Rightarrow \overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,=2-3+4 \\
 & \Rightarrow \overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,=3 \\
\end{align}\]
Hence, 3 is our answer.

Note: One may note that here we have taken the dot product of the two vectors in vector form, you can also use the formula: - \[\overset{\to }{\mathop{a}}\,.\overset{\to }{\mathop{b}}\,=ab\cos \theta \] to get the answer. But this will be a lengthy process as we will have to determine the magnitude of the two vectors and find the cosine of angle between them. You may see that the dot product of the two vectors is a scalar quantity. You must remember one more type of product of two vectors known as cross – product, denotes as \[\overset{\to }{\mathop{a}}\,\times \overset{\to }{\mathop{b}}\,\]. It is a vector product because it results in the formation of a vector which is perpendicular to \[\overset{\to }{\mathop{a}}\,\] and \[\overset{\to }{\mathop{b}}\,\], given as: - \[\overset{\to }{\mathop{a}}\,\times \overset{\to }{\mathop{b}}\,=\left( ab\sin \theta \right)\overset{\wedge }{\mathop{n}}\,\], here \[\overset{\wedge }{\mathop{n}}\,\] is perpendicular to the plane of \[\overset{\to }{\mathop{a}}\,\] and \[\overset{\to }{\mathop{b}}\,\] both.