
If Y-axis is the directrix of the ellipse with eccentricity
Answer
523.2k+ views
Hint- Here, we will proceed by using the formulas for the equation of directrix i.e.,
Complete step by step answer:
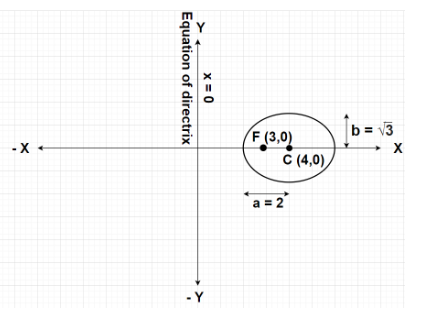
Given, Directrix to the given ellipse is represented by equation of Y-axis i.e., x = 0
Eccentricity,
Focus of the ellipse is at F(3,0)
Since, the directrix of the given ellipse lies along the Y-axis. Therefore, the ellipse will be oriented along the X-axis.
Let the equation of the ellipse along Y-axis is given by
As we know that the equation of the directrix to any ellipse
For the given ellipse, equation of the directrix is x = 0
By putting
Also, the focus coordinates for any ellipse
Also, focus of the given ellipse is F(3,0)
So, h-ae = 3
Hence,
Also, k = 0
Using the formula
Putting a = 2 in equation (2), we get
Putting
This above equation represents the equation of the given ellipse.
Equation of the auxiliary circle to the ellipse
By putting
The above equation represents the required equation of the auxiliary circle to the given ellipse.
Hence, option A is correct.
Note- In this particular problem, firstly it very important to find out that the given ellipse corresponds to which one of the two general cases of ellipse i.e.,
Complete step by step answer:

Given, Directrix to the given ellipse is represented by equation of Y-axis i.e., x = 0
Eccentricity,
Focus of the ellipse is at F(3,0)
Since, the directrix of the given ellipse lies along the Y-axis. Therefore, the ellipse will be oriented along the X-axis.
Let the equation of the ellipse along Y-axis is given by
As we know that the equation of the directrix to any ellipse
For the given ellipse, equation of the directrix is x = 0
By putting
Also, the focus coordinates for any ellipse
Also, focus of the given ellipse is F(3,0)
So, h-ae = 3
Hence,
Also, k = 0
Using the formula
Putting a = 2 in equation (2), we get
Putting
This above equation represents the equation of the given ellipse.
Equation of the auxiliary circle to the ellipse
By putting
The above equation represents the required equation of the auxiliary circle to the given ellipse.
Hence, option A is correct.
Note- In this particular problem, firstly it very important to find out that the given ellipse corresponds to which one of the two general cases of ellipse i.e.,
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Why is the cell called the structural and functional class 12 biology CBSE




