Answer
Verified461.7k+ views
Hint: Draw Venn diagram and fill the details and write the equations in parallel with the information provided in the question. By using a Venn diagram you can find all the values by placing all the given values at the correct place. No language means excluding students reading any subject from all students.
Complete step-by-step answer:
Given that
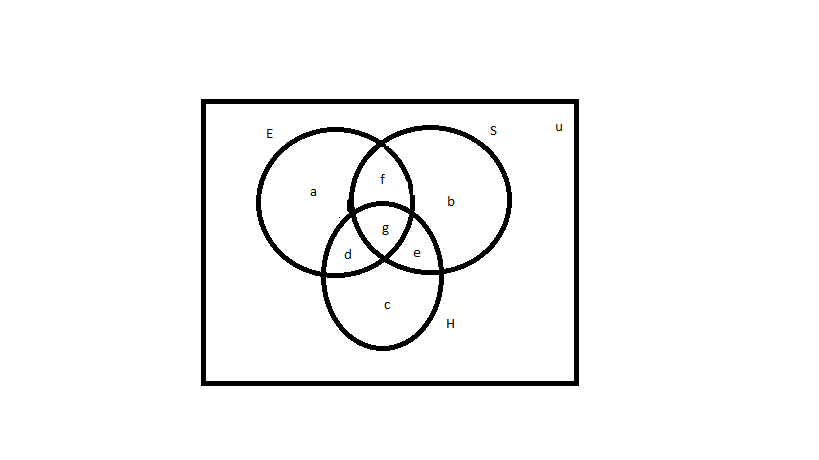
Total number of students in a survey i.e. n(U ) = 100 ,
The number of students studying only English =18 i.e. a = 18
The number of students studying English but not Hindi =23 i.e. a + f = 23
The number of students studying English and Sanskrit i.e. n(E $ \cap $ S) = 8,
The number of students studying English i.e. n(E) =26,
The number of students studying Sanskrit i.e. n(S) = 48 ,
The number of students studying Sanskrit and Hindi i.e. n(S$ \cap $ H) = 8 ,
The number of students who are not studying any language i.e. u – (a+b+c+d+e+f+g) = 24.
Therefore from the given data we get
$ \Rightarrow $ a + d + f + g = 26
$ \Rightarrow $ f + g = 8
$ \Rightarrow $ a = 18
$ \Rightarrow $ 18 + 8 + d = 26
$ \Rightarrow $ d = 0
$ \Rightarrow $ g + e = 8
$ \Rightarrow $ b + f + g + e = 48
$ \Rightarrow $ a + f = 26 – 3 = 23
$ \Rightarrow $ f = 5 , g = 3
$ \Rightarrow $ a + b + c + d + e + f + g = 100 – 24 = 76
$ \Rightarrow $ 66 + c = 76
$ \Rightarrow $ c = 10
from this we can say that d+ g = 0 + 3 = 3.
The number of students who were studying English and Hindi n(E$ \cap $ H) = 3.
Note: While interpreting the question , make sure that the given information is regarding union or intersection. If it is given that both works are done then we should take union and if any of them is done we should take intersection. While solving the question write the equations that you get through the Venn diagram in parallel with solving the problem.
Complete step-by-step answer:
Given that
Total number of students in a survey i.e. n(U ) = 100 ,
The number of students studying only English =18 i.e. a = 18
The number of students studying English but not Hindi =23 i.e. a + f = 23
The number of students studying English and Sanskrit i.e. n(E $ \cap $ S) = 8,
The number of students studying English i.e. n(E) =26,
The number of students studying Sanskrit i.e. n(S) = 48 ,
The number of students studying Sanskrit and Hindi i.e. n(S$ \cap $ H) = 8 ,
The number of students who are not studying any language i.e. u – (a+b+c+d+e+f+g) = 24.
Therefore from the given data we get
$ \Rightarrow $ a + d + f + g = 26
$ \Rightarrow $ f + g = 8
$ \Rightarrow $ a = 18
$ \Rightarrow $ 18 + 8 + d = 26
$ \Rightarrow $ d = 0
$ \Rightarrow $ g + e = 8
$ \Rightarrow $ b + f + g + e = 48
$ \Rightarrow $ a + f = 26 – 3 = 23
$ \Rightarrow $ f = 5 , g = 3
$ \Rightarrow $ a + b + c + d + e + f + g = 100 – 24 = 76
$ \Rightarrow $ 66 + c = 76
$ \Rightarrow $ c = 10
from this we can say that d+ g = 0 + 3 = 3.
The number of students who were studying English and Hindi n(E$ \cap $ H) = 3.
Note: While interpreting the question , make sure that the given information is regarding union or intersection. If it is given that both works are done then we should take union and if any of them is done we should take intersection. While solving the question write the equations that you get through the Venn diagram in parallel with solving the problem.