Question

In $\mathrm{\Delta }ABC$, let L, M, N be the feet of the altitudes. Then calculate $\sin \left(\mathrm{\angle }MLN\right)+\sin \left(\mathrm{\angle }LMN\right)+\sin \left(\mathrm{\angle }MNL\right)$
(a) $4\sin A\sin B\sin C$
(b) $3\sin A\sin B\sin C$
(c) $2\sin A\sin B\sin C$
(d) $\sin A\sin B\sin C$

Answer 1

Hint:After drawing altitude we can join L, M, N to form a pedal triangle and use properties to change angles into A, B, C then use identities $\sin (\pi -\theta )=\sin \theta ,\cos (\pi -\theta )2\cos \theta$
$\sin A+\sin B=2\sin {\displaystyle \frac{(A+B)}{2}}\cos {\displaystyle \frac{(A-B)}{2}}$ and
$\cos A-\cos B=2\sin {\displaystyle \frac{A+B}{2}}\sin \theta {\displaystyle \frac{-A}{2}}$ and get results.

Complete step-by-step answer:
Let’s draw a triangle ABC with altitude AL, BM and CN and then join L, M, N to form a triangle LMN, as shown below:

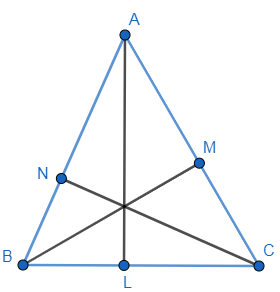
Now we will say triangle LMN is a pedal triangle of triangle ABC. Pedal triangle is a triangle that is obtained by joining points lying on the three sides of the triangle.
So, by using the property of pedal triangle we can write,
i)$\mathrm{\angle }MLN={180}^{\circ }-2\times \mathrm{\angle }A$
ii) $\mathrm{\angle }NML={180}^{\circ }-2\times \mathrm{\angle }B$
iii) $\mathrm{\angle }MLN={180}^{\circ }-2\times \mathrm{\angle }C$
Now we will find what is asked in the equation which is $\sin \left(\mathrm{\angle }MNL\right)+\sin \left(\mathrm{\angle }NML\right)+\sin \left(\mathrm{\angle }MNL\right)$ which can also be written as $\sin ({180}^{\circ }-2\times \mathrm{\angle }A)+\sin ({180}^{\circ }-2\times \mathrm{\angle }B)+\sin ({180}^{\circ }-2\times \mathrm{\angle }C)$
Now we will use the identity,
$\sin ({180}^{\circ }-\theta )=\sin \theta$
So instead of $\theta$ we will put values 2A, 2B, 2C so,
$\sin \left(\mathrm{\angle }MNL\right)+\sin \left(\mathrm{\angle }NML\right)+\sin \left(\mathrm{\angle }MNL\right)=\sin \left(2A\right)+\sin \left(2B\right)+\sin \left(2C\right)$
As we know that in triangle ABC we can say $A+B+C={180}^{\circ }$,
Then we can write,
$\sin \left(2A\right)+\sin \left(2B\right)+\sin \left(2C\right)$ as $2\sin A\cos A+2\sin (B+C)\cos (B-C)$
Using formula $\sin 2\theta =2\sin \theta \cos \theta$ . Where $\theta$ can be replaced by A and by using another formula,
$\sin C+\sin D=2\sin {\displaystyle \frac{(C+D)}{2}}\cos {\displaystyle \frac{(C-D)}{2}}$where C can be taken as 2B and D can be taken as 2C.
So, $2\sin A\cos A+2\sin (B+C)\cos (B-C)$
Now as we know that $A+B+C=\pi$ then we can write $B+C=\pi -A$
So,$2\sin A\cos A+2\sin (\pi -A)\cos (B-C)$
Now we will use the identity $\sin (\pi -\theta )-\sin \theta$ so in place of $\theta$ we will use A.
Now we can write it as,
$2\sin A\cos A+2\mathrm{sinA}\cos (B-C)$ we take common $2\sin A$ from both the terms so we can write it as,
$2\sin A(\cos A+\cos (B-C))$
Now we will substitute A as $\pi -(B+C)$ then $\cos (\pi -(B+C))=-\cos (B+C)$
Using identity $\cos (\pi -\theta )=-\cos \theta$
$2\sin A(-\cos (B+C)+\cos (B-C))$
So by rearranging and writing it as,
$2\sin A(\cos (B-C)-\cos (B+C))$
Now we will use identity that,
$\cos (B-C)-\cos (B+C)=2\sin B\sin C$
Hence we can write it as,
$2\sin A(2\sin B\sin C)=4\sin A\sin B\sin C$
Hence the correct option is ‘A’.

Note: Students should know all the trigonometric formulas and identities before solving the problem related like this. They should know the facts about pedal triangles too.
Students often make mistakes when considering the pedal triangle property.