
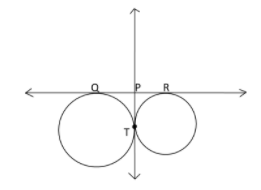
In figure, QR is a common tangent to the given circles, touching externally at the point T. The tangent at T meets QR at P. If $PT = 3.8cm$, then find the length of QR.

Answer
495.9k+ views
Hint: Observe the tangents drawn to both the circles. Use the theorem, the lengths of tangents drawn from an external point to a circle are equal. You will obtain $QR = 2PT$.
Complete step-by-step answer:
Two circles can be seen in the figure. For better understanding, lets name the bigger circle ${C_1}$and the smaller circle ${C_2}$.
We can see that tangents PQ and PT are drawn from an external point P to the circle ${C_1}$.
So,
$PQ = PT$ ---(1)
{Lengths of tangents drawn from an external point to a circle are equal.}
Similarly,
Tangents PR and PT are drawn to the circle ${C_2}$from an external point P;
Again using the same theorem,
$PR = PT$ ----(2)
QR can be written as
$QR = QP + PR$ ---(3)
Substituting the values of PQ and PR from equations (1) and (2) respectively, in equation (3),
We obtain,
$
QR = PT + PT \\
QR = 2PT \\
$
Given that $PT = 3.8cm$
So,
$
QR = 2 \times 3.8 \\
QR = 7.6cm \\
$
Note: It should be noted that in such type of questions, one must not confuse tangent and secant; a tangent to a circle is a straight line in the plane of circle, which touches the circle at only one point whereas secant to a circle intersects it at two distinct point. The theorems of tangent to a circle should not be applied on a secant as the results will be wrong.
Complete step-by-step answer:

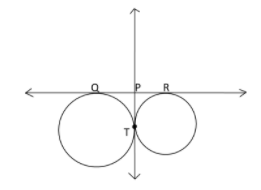
Two circles can be seen in the figure. For better understanding, lets name the bigger circle ${C_1}$and the smaller circle ${C_2}$.
We can see that tangents PQ and PT are drawn from an external point P to the circle ${C_1}$.
So,
$PQ = PT$ ---(1)
{Lengths of tangents drawn from an external point to a circle are equal.}
Similarly,
Tangents PR and PT are drawn to the circle ${C_2}$from an external point P;
Again using the same theorem,
$PR = PT$ ----(2)
QR can be written as
$QR = QP + PR$ ---(3)
Substituting the values of PQ and PR from equations (1) and (2) respectively, in equation (3),
We obtain,
$
QR = PT + PT \\
QR = 2PT \\
$
Given that $PT = 3.8cm$
So,
$
QR = 2 \times 3.8 \\
QR = 7.6cm \\
$
Note: It should be noted that in such type of questions, one must not confuse tangent and secant; a tangent to a circle is a straight line in the plane of circle, which touches the circle at only one point whereas secant to a circle intersects it at two distinct point. The theorems of tangent to a circle should not be applied on a secant as the results will be wrong.
Recently Updated Pages
Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Trending doubts
A number is chosen from 1 to 20 Find the probabili-class-10-maths-CBSE

Find the area of the minor segment of a circle of radius class 10 maths CBSE

Distinguish between the reserved forests and protected class 10 biology CBSE

A boat goes 24 km upstream and 28 km downstream in class 10 maths CBSE

A gulab jamun contains sugar syrup up to about 30 of class 10 maths CBSE

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE




