Question

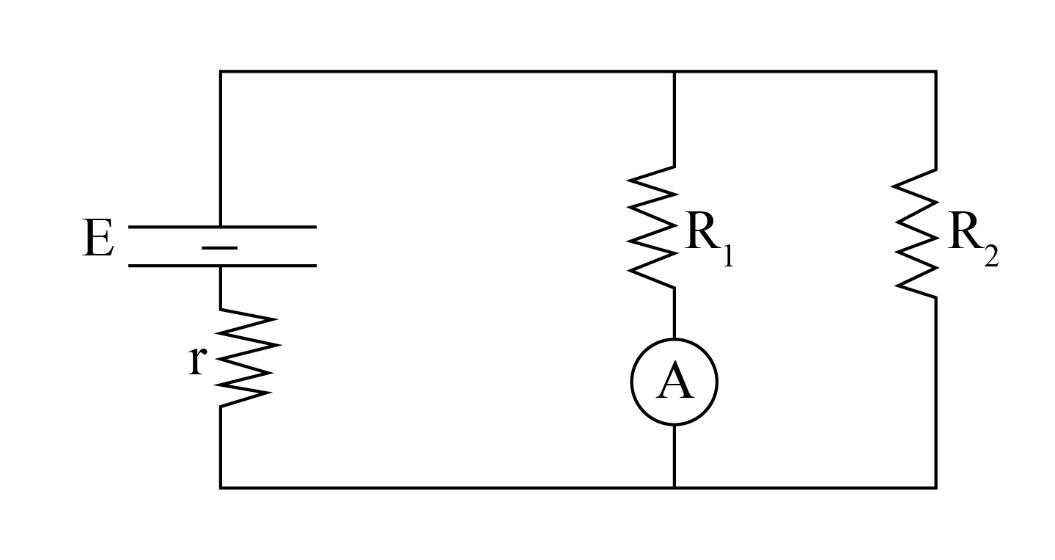
In the circuit shown below, the ammeter reading will be independent of \[{R_2}\] when

A. \[{R_1} + {R_2} = r\]
B. \[{R_1} = 2r\]
C. \[{R_1} - {R_2} = r\]
D. \[r = 0\]

Answer 1

Hint: We will reduce the given circuit by using equivalent resistance expression when two resistors are in series or parallel combination. We will also establish the relationship between ammeter current and current through the reduced circuit to find the final expression for ammeter reading.

Complete step by step answer:
By closely observing the given circuit, we see that the two resistors of resistances are parallel combinations. Therefore, using the concept of equivalent resistance of two resistors when they are connected in parallel combination, we can write:
\[R' = \dfrac{{{R_1}{R_2}}}{{{R_1} + {R_2}}}\]
Again observing the circuit closely, we will find that the equivalent resistance of the given is equal to the summation of resistance R’ and r because they are connected in series combination so we can write:
\[{R_{eq}} = R' + r\]
On substituting \[\dfrac{{{R_1}{R_2}}}{{{R_1} + {R_2}}}\] for R’ in the above expression to get the value of equivalent resistance, we get:
\[{R_{eq}} = \dfrac{{{R_1}{R_2}}}{{{R_1} + {R_2}}} + r\]
On expanding the above term, we can write:
\[{R_{eq}} = \dfrac{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}{{{R_1} + {R_2}}}\]
We can write the expression for current flowing through the reduced circuit as below:
\[I = \dfrac{E}{{{R_{eq}}}}\]
On substituting \[\dfrac{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}{{{R_1} + {R_2}}}\] for \[{R_{eq}}\] in the above expression, we can write:
\[\begin{array}{c}
I = \dfrac{E}{{\dfrac{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}{{{R_1} + {R_2}}}}}\\
 = \dfrac{{E\left( {{R_1} + {R_2}} \right)}}{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}
\end{array}\]
We know that the ammeter reading is the value of current through it, and it can be expressed as:
\[{I_1} = \dfrac{{{R_2}}}{{{R_1} + {R_2}}}I\]
Here \[{I_1}\] is the ammeter current.
On substituting \[\dfrac{{E\left( {{R_1} + {R_2}} \right)}}{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}\] for I in the above expression, we get:
\[\begin{array}{c}
{I_1} = \dfrac{{{R_2}}}{{{R_1} + {R_2}}}\left[ {\dfrac{{E\left( {{R_1} + {R_2}} \right)}}{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}} \right]\\
 = \dfrac{{E{R_2}}}{{{R_1}{R_2} + r\left( {{R_1} + {R_2}} \right)}}
\end{array}\]
On dividing the right-hand side by \[{R_2}\], we get:
\[{I_1} = \dfrac{E}{{{R_1} + r\left( {\dfrac{{{R_1}}}{{{R_2}}} + 1} \right)}}\]
On closely observing the above equation, we can conclude that if r is equal to zero, then the ammeter reading \[{I_1}\] will become independent of \[{R_2}\].
Therefore, we can say that the ammeter reading will be independent of \[{R_2}\] when r is equal to zero

So, the correct answer is “Option D”.

Note:
We have to note that the ammeter is used to measure current, so ammeter reading is nothing but the current through it. We also know that current in the circuit is divided, so ammeter current and current through the reduced circuit have different values.