Question

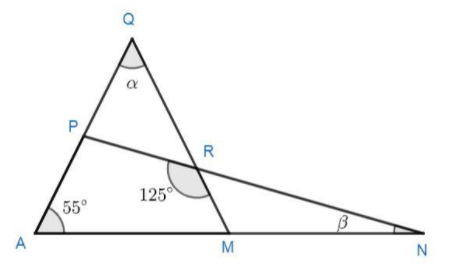
In the diagram AMN, QRM and PRN are all straight lines. The value of $\alpha +\beta$ is

Answer 1

Hint: Use the property of the triangle that the sum of the angles of the triangle is ${180}^{\circ }$in the triangle AQM, it gives:
$$\mathrm{\angle }AQM+\mathrm{\angle }QAM+\mathrm{\angle }AMQ={180}^{\circ }$$
After that use the property of the sum of the linear pairs of angles, which says that if we have a pair of linear angles then their sum is${180}^{\circ }$.

Complete step by step solution:
It is given in the problem that AMN, QRM, and PRN are all straight lines and we have to find the value of$\left(\alpha +\beta \right)$.
First, applying the angle sum property in triangle AQM,
We know that the sum of the angles in a triangle is always${180}^{\circ }$. That is,
$$\mathrm{\angle }AQM+\mathrm{\angle }QAM+\mathrm{\angle }AMQ={180}^{\circ }$$
Substitute the values of the given angles$\mathrm{\angle }AQM=\alpha$and$\mathrm{\angle }QAM={55}^{\circ }$into the equation.
$$\alpha +{55}^{\circ }+\mathrm{\angle }AMQ={180}^{\circ }$$
$$\mathrm{\angle }AMQ={180}^{\circ }-{55}^{\circ }-\alpha$$
On simplifying the above equation, we get
$$\mathrm{\angle }AMQ={125}^{\circ }-\alpha$$
As we have given that AMN is a straight line, we know that the linear pair of angles have the sum${180}^{\circ }$, then
$\mathrm{\angle }$AMQ + $\mathrm{\angle }$RMN = 180°
Now, substitute the values into the equation:
$${125}^{\circ }-\alpha +\mathrm{\angle }RMN={180}^{\circ }$$
$$\mathrm{\angle }RMN={55}^{\circ }+\alpha$$
It is also given to us in the problem that PRN is a straight line, so we know that the linear pair of angles have the sum ${180}^{\circ }$, then
$$\mathrm{\angle }PRM+\mathrm{\angle }MRN={180}^{\circ }$$
$${125}^{\circ }+\mathrm{\angle }MRN={180}^{\circ }$$
$$\mathrm{\angle }MRN={55}^{\circ }$$
We again use the property of the sum of the angles of the triangle MRN. Then we have,
$$\mathrm{\angle }MRN+\mathrm{\angle }RMN+\mathrm{\angle }RNM={180}^{\circ }$$
Substitute the values into the equation:
$${55}^{\circ }+{55}^{\circ }+\alpha +\beta ={180}^{\circ }$$
$$\alpha +\beta ={70}^{\circ }$$

$\therefore$The required sum of angles have the value$$\alpha +\beta ={70}^{\circ }$$.

Note:
Try to find all the angles of the triangle RMN in terms of $\alpha$using the angle sum property and linear pair of angles property of the triangle.