
In the given graph. The slope of line of AB gives the information of the.
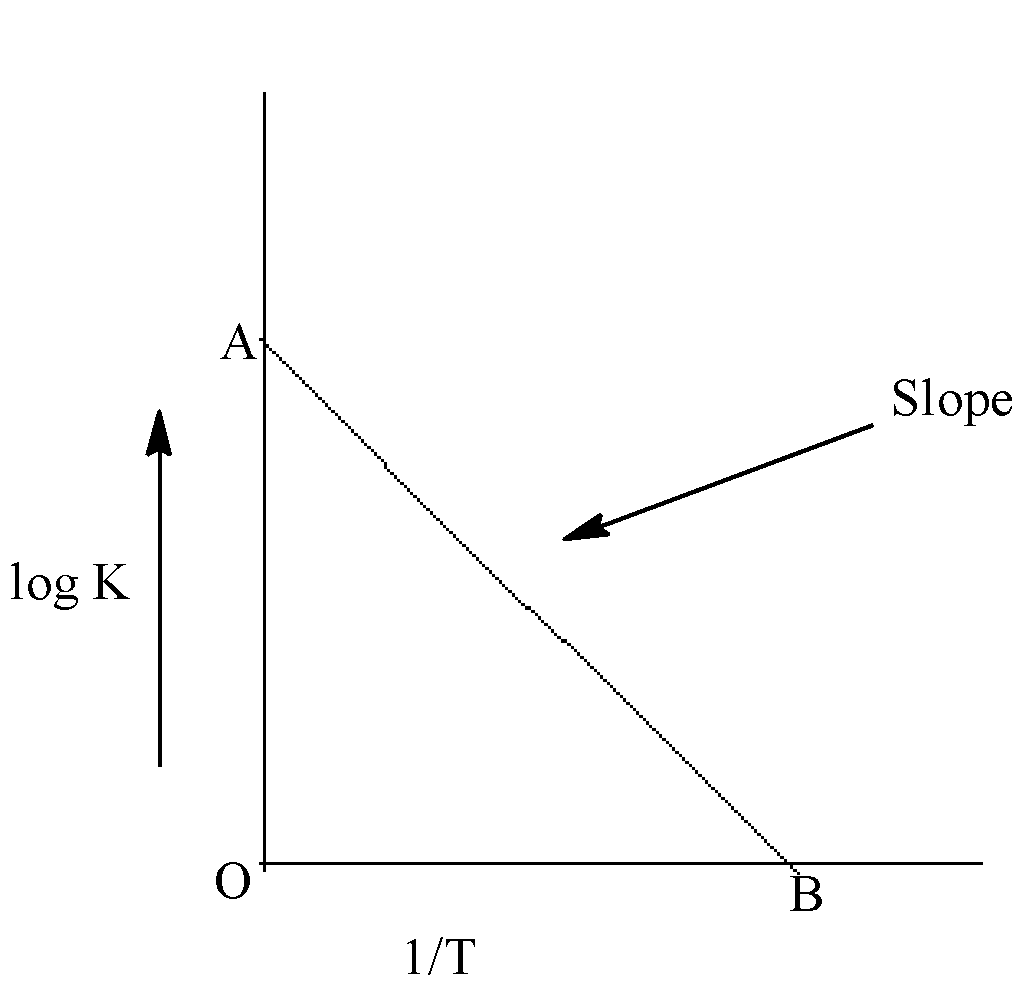
A) Value of
B) Value of
C) Value of
D) Value of

Answer
493.5k+ views
Hint: We Know the Arrhenius equation,
The Arrhenius equation is
The rate constant of the reaction is K.
The activation energy of the reaction is
R is the gas constant of the reaction
We must remember that the frequency factor or Arrhenius factor explains the rate of collision and the fraction of collisions with the proper orientation for the reaction to occur and it is denoted as
Complete step by step answer:
Now we take the natural logarithm on both sides of Arrhenius equation we get,
Thus,
So, the correct answer is Option C .
Note:
Let us discuss the concept of activation energy.
The difference between the energy state of reactants and therefore the transition state for the reaction to happen reactants got to cross the transition state energy barrier and hence lower energy of activation faster is going to be the reaction.
One can calculate the activation energy if two known temperatures are directly given and a rate constant at each temperature is known using the equation.
Where
Example:
The activation energy of a reaction when its rate is double if the temperature is raised from
Hence the rate of the reaction is doubled on raising the temperature, thus the rate of the reaction is,
We know that the rate of the reactions and the reactions rate are proportional to each other,
Substituting the know values in the equation,
On simplifying we get,
The activation energy for a reaction is
The Arrhenius equation is
The rate constant of the reaction is K.
The activation energy of the reaction is
R is the gas constant of the reaction
We must remember that the frequency factor or Arrhenius factor explains the rate of collision and the fraction of collisions with the proper orientation for the reaction to occur and it is denoted as
Complete step by step answer:
Now we take the natural logarithm on both sides of Arrhenius equation we get,
Thus,
So, the correct answer is Option C .
Note:
Let us discuss the concept of activation energy.
The difference between the energy state of reactants and therefore the transition state for the reaction to happen reactants got to cross the transition state energy barrier and hence lower energy of activation faster is going to be the reaction.
One can calculate the activation energy if two known temperatures are directly given and a rate constant at each temperature is known using the equation.
Where
Example:
The activation energy of a reaction when its rate is double if the temperature is raised from
Hence the rate of the reaction is doubled on raising the temperature, thus the rate of the reaction is,
We know that the rate of the reactions and the reactions rate are proportional to each other,
Substituting the know values in the equation,
On simplifying we get,
The activation energy for a reaction is
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Economics: Engaging Questions & Answers for Success

Trending doubts
Draw a labelled sketch of the human eye class 12 physics CBSE

a Tabulate the differences in the characteristics of class 12 chemistry CBSE

Which one of the following is a true fish A Jellyfish class 12 biology CBSE

Why is the cell called the structural and functional class 12 biology CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Write the difference between solid liquid and gas class 12 chemistry CBSE




